
A person is unable to see objects distinctly placed within
Answer
488.4k+ views
Hint: We know about four types of vision defects i.e. hypermetropia, myopia, presbyopia, and astigmatism. Two of the among are the problem of seeing an object in different places. Such as, for Hypermetropia we can’t see the nearer object, and for Myopia we can't see the far objects.
These two diseases happen for the small or big size of the eyeball than its natural size and also for the increase or the decrease of the focal length of the eye lenses. To calculate the power use the general lens equation and the relation between the power and the focal length.
Formula used:
Where
The power of the lens,
Complete step by step solution:
A person is unable to see objects distinctly placed within
The two possible reasons for the defect is :
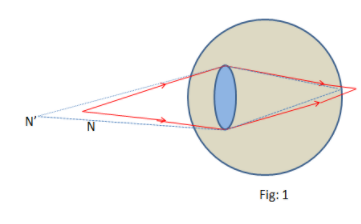
Let, the point
From the lens equation
Where
Given,
Since,
The power of the convex lens,
Since,
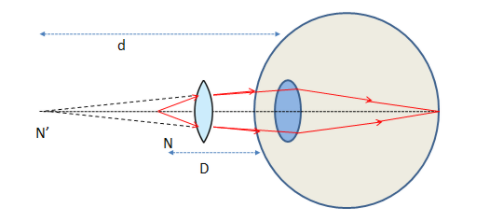
Note: The power of the convex lens is positive since the focal length is positive. Hence to correct the Hypermetropia or the Long sight defect a person should use the spectacles of a convex lens or we may say he should use spectacles of positive power.
These two diseases happen for the small or big size of the eyeball than its natural size and also for the increase or the decrease of the focal length of the eye lenses. To calculate the power use the general lens equation and the relation between the power and the focal length.
Formula used:
Where
The power of the lens,
Complete step by step solution:
A person is unable to see objects distinctly placed within
The two possible reasons for the defect is :

Let, the point
From the lens equation
Where
Given,
Since,
The power of the convex lens,
Since,

Note: The power of the convex lens is positive since the focal length is positive. Hence to correct the Hypermetropia or the Long sight defect a person should use the spectacles of a convex lens or we may say he should use spectacles of positive power.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




