Question

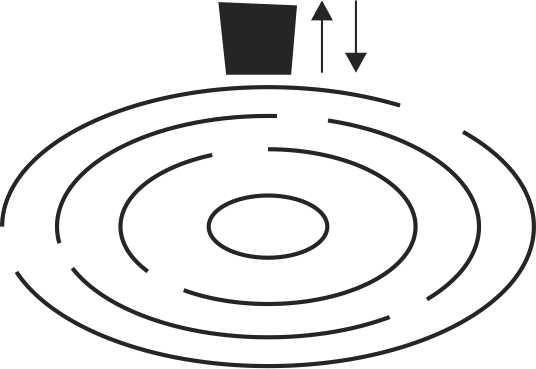
A piece of cork is floating on water in a small tank. The cork oscillates up and down vertically when small ripples pass over the surface of water. The velocity of the ripples being $0.21\;{\text{m}}{{\text{s}}^{ - 1}}$, wave length \[15{\text{ mm}}\] and amplitude \[5{\text{ mm}}\], the maximum velocity of the piece of cork is $\left( {\pi = \dfrac{{22}}{7}} \right)$.

Answer 1

Hint: In this problem, first calculate the frequency of the cork by substituting the given values in the formula and then by using the calculated value of the frequency, find the maximum velocity of the cork.

Complete step-by-step solution:
As we know that the angular frequency is a frequency of a steadily recurring phenomenon, and it is expressed in radians per second.
The formula to calculate the angular frequency is given by, $\omega = 2\pi f$.
Here, $f$ is a linear frequency. We can define the linear frequency as the number of complete oscillations in each period, and it is expressed in hertz.
Now, substitute $2\pi f$ for $\omega $ in the equation ${v_{\max }} = \omega A$ to get the formula for ${v_{\max }}$ as,
${v_{\max }} = 2\pi fA$
Here, $A$ is the amplitude and it can be defined as the maximum extent of a vibration or oscillation, measured from the position of equilibrium and it is expressed in mm or m.
The formula to calculate the linear frequency is given by, $f = \dfrac{u}{\lambda }$.
Here, $\lambda $ is the wavelength which is defined as the distance measured between repeated crests of a wave, especially in case of a sound wave or electromagnetic wave, and $u$ is the velocity of ripples.
In the given problem, velocity of the ripple is $0.21\;{\text{m}}{{\text{s}}^{ - 1}}$, wavelength is \[15{\text{ mm}}\], and amplitude is \[5{\text{ mm}}\].
Now, find the linear frequency of the piece of cork by substituting $0.21\;{\text{m}}{{\text{s}}^{ - 1}}$ for $u$, and $0.015\;{\text{m}}$ for $\lambda $ in the formula as,
$
  f = \dfrac{u}{\lambda } \\
    = \dfrac{{0.21}}{{0.015}} \\
    = 14\;{\text{Hz}} \\
 $
Similarly, find the maximum velocity of the piece of cork by Substituting $\dfrac{{22}}{7}$ for $\pi $, $14\;{\text{Hz}}$ for $f$, and $0.005\;{\text{m}}$ for $A$ in the formula ${v_{\max }} = 2\pi fA$.
$
  {v_{\max }} = 2\pi fA \\
   = 2\left( {\dfrac{{22}}{7}} \right)\left( {14} \right)\left( {0.005} \right) \\
   = 0.44\;{\text{m/s}} \\
 $
Therefore, the maximum velocity of the piece of cork is $0.44\;{\text{m/s}}$.

Note:- Make sure that the maximum velocity of the piece of cork is the product of angular frequency, and amplitude where angular frequency is given by $\omega = 2\pi f$, and use the formula $f = \dfrac{u}{\lambda }$ to find the linear frequency.