
A plano-convex lens, when silvered on the plane side, behaves like a concave mirror of focal length 60cm. However, when silver on the convex side, it behaves like a concave mirror of focal length 20cm. Then, the refractive index of the lens is:
A. 3.0
B. 1.5
C. 1.0
D. 2.0
Answer
439.1k+ views
3 likes
Hint: We can consider the plano-convex lens with plane-side mirrored to act like two lenses. Use the formula for two consecutive lenses to find the effective focal length of the convex part. Then find the Radius of curvature using the second condition. Finally, use the focal length formula of the plano-convex lens to find the final result.
Formula Used:
The effective focal length of a plano-convex or plano-concave or similar mirrors is given by,
Focal length formula of the plano-convex lens is given by,
Where,
Complete step-by-step answer:
First, let us look at the first configuration.
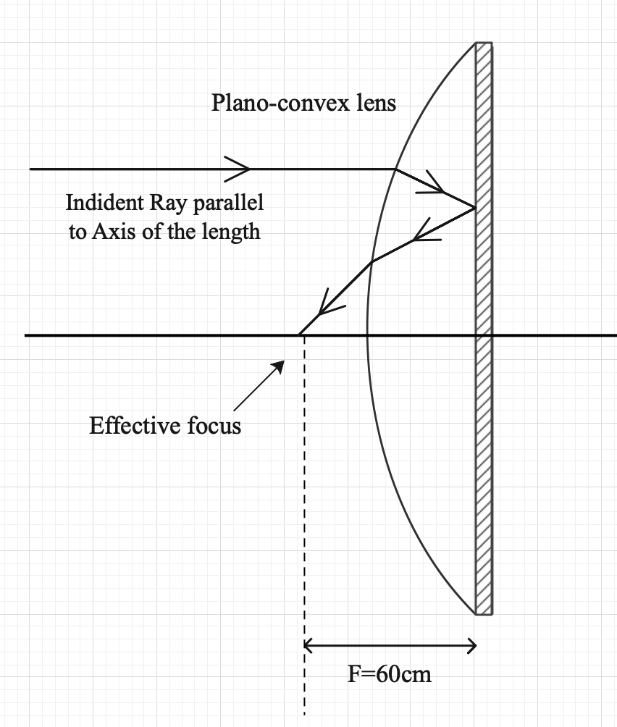
From equation (1) we can write that,
Given that,
So, we write,
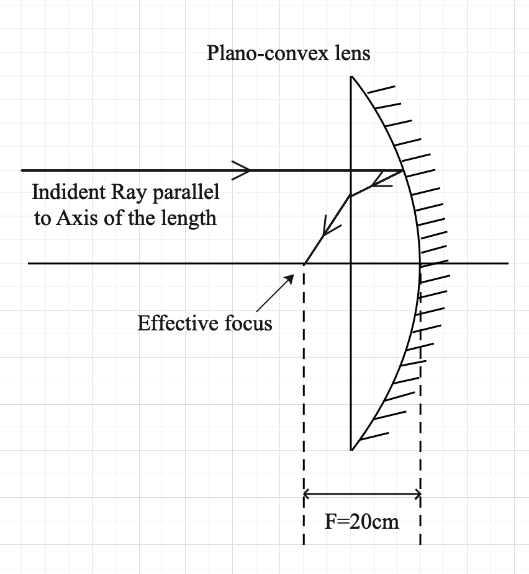
Now, for the second case,
Here, the convex side is mirrored, so
And the overall system works like a concave mirror of focal length 20 cm.
Hence,
So, we can write,
Hence, R =60cm
So, we can use equation (2) to find the refractive index of the lens. We can write,
Hence, refractive index of the lens =1.5
So, the correct answer is - (B)
Note:
These equations should be memorized for accurate and fast solutions. However, you can follow the ray to find the image due to each refraction and reflection to find the focal length. However, that process is tough and time-consuming.
Formula Used:
The effective focal length of a plano-convex or plano-concave or similar mirrors is given by,
Focal length formula of the plano-convex lens is given by,
Where,
Complete step-by-step answer:
First, let us look at the first configuration.

From equation (1) we can write that,
Given that,
So, we write,

Now, for the second case,
Here, the convex side is mirrored, so
And the overall system works like a concave mirror of focal length 20 cm.
Hence,
So, we can write,
Hence, R =60cm
So, we can use equation (2) to find the refractive index of the lens. We can write,
Hence, refractive index of the lens =1.5
So, the correct answer is - (B)
Note:
These equations should be memorized for accurate and fast solutions. However, you can follow the ray to find the image due to each refraction and reflection to find the focal length. However, that process is tough and time-consuming.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




