Question

A point object is placed midway between two plane mirrors at distance ‘a’ apart. The plane mirror forms an infinite number of images due to multiple reflections. The distance between the first two images formed behind any mirror is:
$\left( A \right)$ a
$\left( B \right)$ 2a
$\left( C \right)$ a/2
$\left( D \right)$ 4a

Answer 1

Hint: When we place any object mid-way between the two plane mirrors the distance of the image formed behind any mirror is the same as the distance by which the mirror is apart so use this concept to reach the solution of the question.

Complete step-by-step solution -

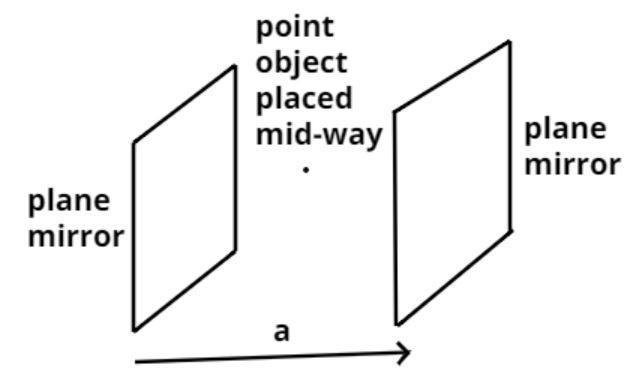
The pictorial representation of the given problem is shown above.
The two mirrors are placed apart by distance ‘a’, as shown in the figure.
A point object is placed midway between the two plane mirrors as shown in the figure.
So there are multiple images formed due to reflections and the distance of these images from the mirror w.r.t another mirror is same for the first two images by the distance by which the mirror is apart because for the first two images they are formed exactly parallel to the point object so the distance of the first two images is same by which the mirror is apart.
So the distance between the images formed = a.
So this is the required answer.
Hence option (A) is the correct answer.

Note – Whenever we face such types of questions always remember that if we place the object midway between the two plane mirrors the first two images are formed exactly parallel to the object and the distance between two images w.r.t the mirrors are the same by which the mirror is apart and the rest of the images are not parallel to each other so the distances of the rest of the images is not the same by which the mirror is apart.