
A point P moves so that its perpendicular distance from two given lines
Answer
518.7k+ views
Hint:Try to imagine the way
Complete step-by-step answer:
Now let’s try imagining the condition. For that, first we’ll consider the case where the lines given are parallel to each other and never intersect. Now, for the point to be equidistant from both the lines, it is necessary that it remains so at each point of its locus. So, we can assume two perpendicularly opposite points on the two parallel lines. Now, we need to find another point between these two assumed points, that is equidistant to them both, and this will be none other than the two points’ midpoint. Therefore, if we go on extrapolating the individual midpoints of each pair of opposite points that we assume on the parallel lines, we’ll ultimately arrive at a straight line, that is midway between the two lines, and is parallel to them both.
Thus, when two lines
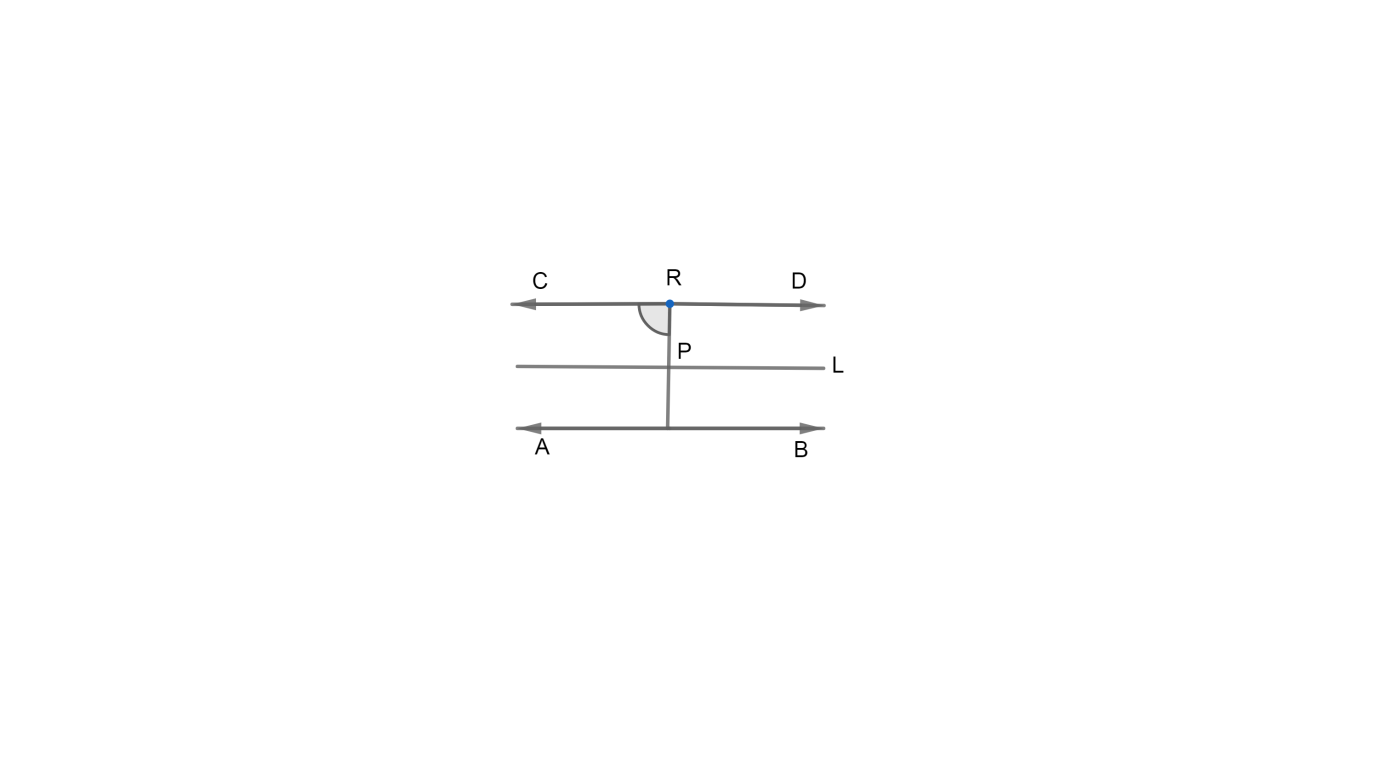
Now, let’s imagine the condition where the lines given are intersecting. For this, let’s assume the case where the point is equidistant to both the lines, and the distance is zero. This is possible only in one condition, when the point lies at the intersection of the two lines. Hence, one point in the locus of the point will actually be the intersection point of the two lines. Now, try imagining what will happen if we move along the angle bisector of the angle formed by the intersecting lines. Below is a figure to aid you in imagining.
If
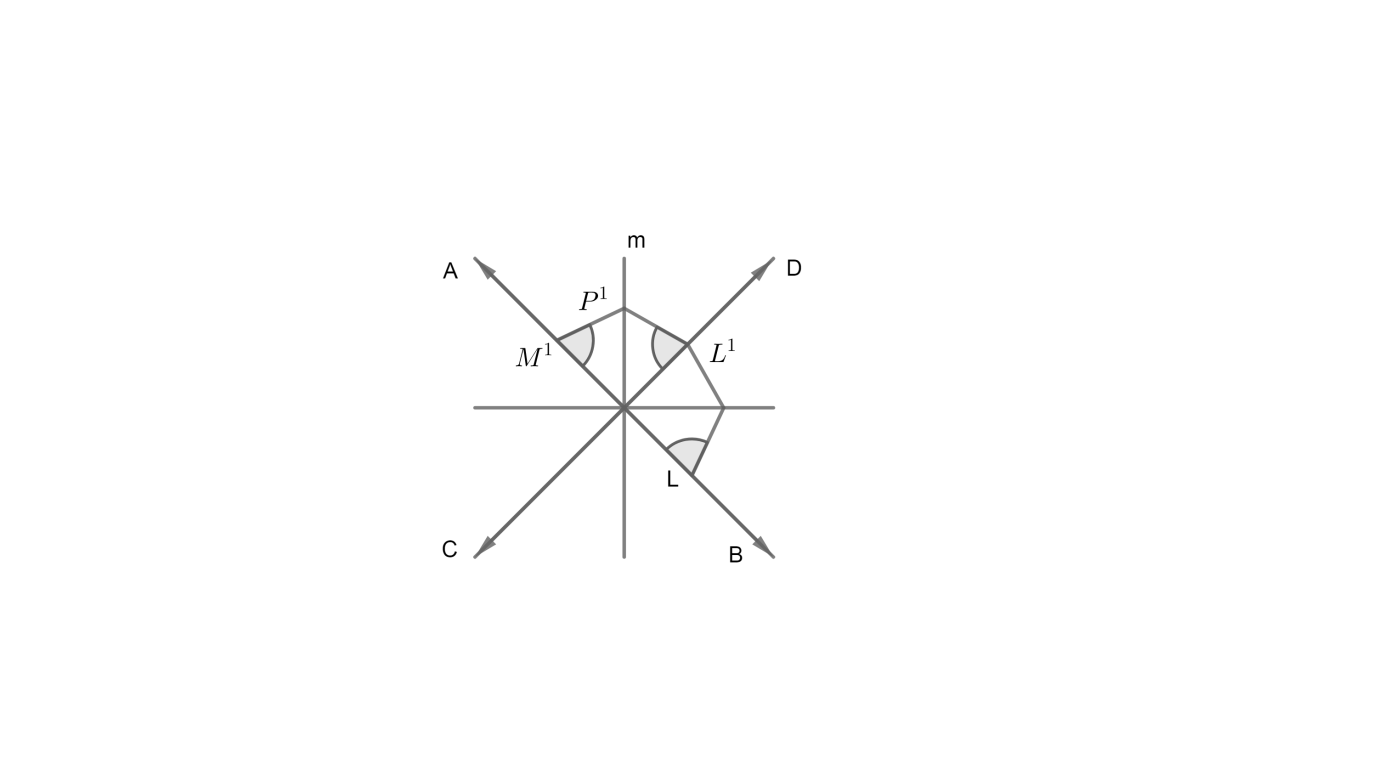
Thus, the locus of point
Note: Most of the students often misunderstand the concept of
Complete step-by-step answer:
Now let’s try imagining the condition. For that, first we’ll consider the case where the lines given are parallel to each other and never intersect. Now, for the point to be equidistant from both the lines, it is necessary that it remains so at each point of its locus. So, we can assume two perpendicularly opposite points on the two parallel lines. Now, we need to find another point between these two assumed points, that is equidistant to them both, and this will be none other than the two points’ midpoint. Therefore, if we go on extrapolating the individual midpoints of each pair of opposite points that we assume on the parallel lines, we’ll ultimately arrive at a straight line, that is midway between the two lines, and is parallel to them both.
Thus, when two lines

Now, let’s imagine the condition where the lines given are intersecting. For this, let’s assume the case where the point is equidistant to both the lines, and the distance is zero. This is possible only in one condition, when the point lies at the intersection of the two lines. Hence, one point in the locus of the point will actually be the intersection point of the two lines. Now, try imagining what will happen if we move along the angle bisector of the angle formed by the intersecting lines. Below is a figure to aid you in imagining.
If

Thus, the locus of point
Note: Most of the students often misunderstand the concept of
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




