
A ray incident parallel to principal axis on a concave mirror at an angle
Answer
483.6k+ views
Hint:As the ray becomes parallel after two reflections, so the sum of all the angles it made on the same side of the parallel lines is 180°.The centre of the reflecting surface of a sphere of which a spherical mirror is made up of is called the centre of curvature of the spherical mirror. The centre of curvature of a concave mirror lies in front of it.
Complete step by step answer:
Let a ray parallel to the principal axis PQ meet the mirror at A as shown in the figure. After reflection from the surface it meets at B. After reflection from B it emerges parallel to the principal axis. It is given that
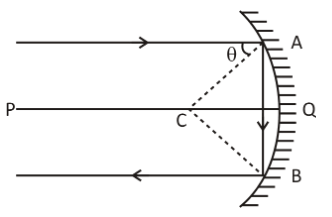
As the ray incident parallel to the principal axis on a concave mirror at an angle after two reflections, reflected ray becomes parallel to incident ray. So taking the centre of curvature as reference(refer the following figure), the sum of the interior opposite angles on the same side of parallel lines is 180°.
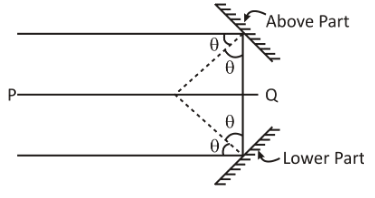
Therefore, we have
Comparing it with the equation
Hence, the value of N is 4.
Note:In a concave mirror, a ray parallel to the principal axis, after reflection, will pass through the principal focus. In case of a convex mirror it appears to diverge from the principal focus. A ray passing through the principal focus of a concave mirror after reflection, will emerge parallel to the principal axis. So after first reflection the given ray will pass through the principal focus and after second reflection, it will emerge parallel to the principal axis.
Complete step by step answer:
Let a ray parallel to the principal axis PQ meet the mirror at A as shown in the figure. After reflection from the surface it meets at B. After reflection from B it emerges parallel to the principal axis. It is given that

As the ray incident parallel to the principal axis on a concave mirror at an angle after two reflections, reflected ray becomes parallel to incident ray. So taking the centre of curvature as reference(refer the following figure), the sum of the interior opposite angles on the same side of parallel lines is 180°.

Therefore, we have
Comparing it with the equation
Hence, the value of N is 4.
Note:In a concave mirror, a ray parallel to the principal axis, after reflection, will pass through the principal focus. In case of a convex mirror it appears to diverge from the principal focus. A ray passing through the principal focus of a concave mirror after reflection, will emerge parallel to the principal axis. So after first reflection the given ray will pass through the principal focus and after second reflection, it will emerge parallel to the principal axis.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




