
A rectangular coil of sides
Answer
506.1k+ views
Hint: First find the area of cross-section of the coil. Then find a magnetic moment associated with the coil. From the value of the magnetic moment, calculate the torque (rotating force) acting one the dipole.
Formula used: Torque,
Magnetic moment of the current loop,
Force on a current carrying conductor of length
Where
Torque,
Complete step by step answer:
Area of the coil,
Magnetic moment associated with the coil is,
Here
The torque acting on the coil is given by
For the given case
Here the angle between the normal and the magnetic field is
So, Torque,
Additional Information: Fleming’s left hand rule states that when a current carrying conductor is placed in an external magnetic field it experiences a force which is perpendicular to the plane containing both magnetic field and flow of current. i.e. if you place your thumb, index finger and middle finger perpendicular to each other. And if the index finger represents the direction of the applied field, the middle finger represents the direction of flow of current, then the thumb will represent the force on the conductor. So the conductor will experience a force in upward direction and will try to move in that direction.
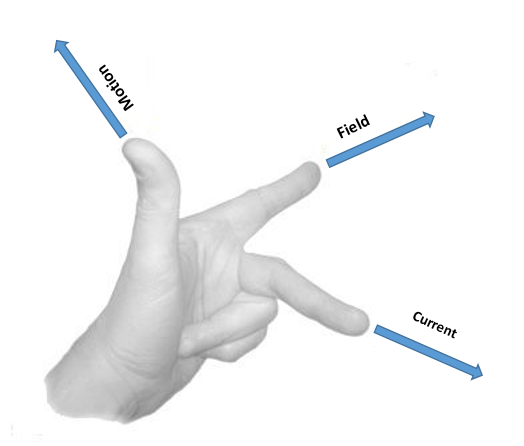
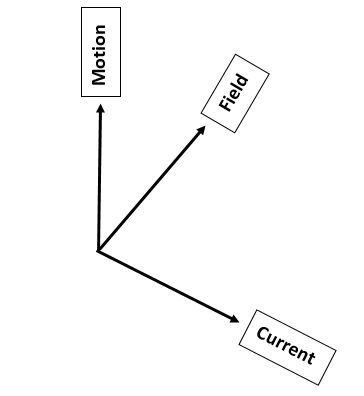
Note: The expression
A planar current loop of a given perimeter suspended in a magnetic field will experience maximum torque when the shape of the loop is circular, because a given perimeter circle has maximum area.
Formula used: Torque,
Magnetic moment of the current loop,
Force on a current carrying conductor of length
Where
Torque,
Complete step by step answer:
Area of the coil,
Magnetic moment associated with the coil is,
Here
The torque acting on the coil is given by
For the given case
Here the angle between the normal and the magnetic field is
So, Torque,
Additional Information: Fleming’s left hand rule states that when a current carrying conductor is placed in an external magnetic field it experiences a force which is perpendicular to the plane containing both magnetic field and flow of current. i.e. if you place your thumb, index finger and middle finger perpendicular to each other. And if the index finger represents the direction of the applied field, the middle finger represents the direction of flow of current, then the thumb will represent the force on the conductor. So the conductor will experience a force in upward direction and will try to move in that direction.


Note: The expression
A planar current loop of a given perimeter suspended in a magnetic field will experience maximum torque when the shape of the loop is circular, because a given perimeter circle has maximum area.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




