
A rectangular park is 100 m by 50 m. It is surrounded by semi-circular flower beds all around. Find the cost of levelling the semi-circular flower beds at 60 paisa per square metre (Use
Answer
535.5k+ views
Hint- Here, we will be proceeding by calculating the total area of semi-circular flower beds using the formula of area of a semi-circle which is given by
Complete step-by-step answer:
Given, Length of the rectangular park= 100 m
Breadth of the rectangular park= 50 m
Since, 1 paisa = Rs
Rate of levelling the semi-circular flower beds= 60 paisa per
The cost of levelling the semi-circular flower beds which are there around the rectangular park is required. This cost of levelling depends upon the total area of the semi-circular flower beds.
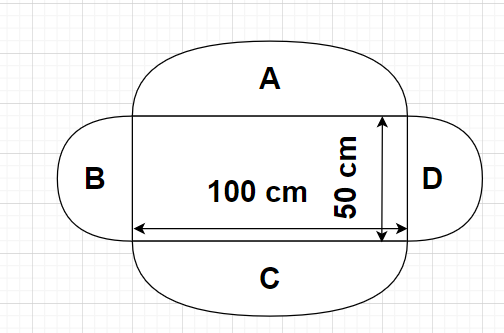
Clearly, from the figure we can see that there are a total four semi-circular flower beds around the rectangular park out of which two of them (A and C) have equal diameters and the other two (B and D) also have equal diameters. If diameters are equal that means radius is also equal and hence areas will also be equal.
From the figure, Diameter of semi-circular flower bed A = Length of rectangular park = 100 m
As we know that, Radius of a circle
So, Radius of semi-circular flower bed A
Also we know that, Area of a semi-circle with a radius R
Area of semi-circular flower bed A
Since, semi-circular flower beds A and C will be having equal diameters and hence equal areas.
So, Area of semi-circular flower bed C = 3925
Again from the figure, Diameter of semi-circular flower bed B = Breadth of rectangular park = 50 m
As we know that, Radius of a circle
So, Radius of semi-circular flower bed B
Also we know that, Area of a semi-circle with a radius R
Area of semi-circular flower bed B
Since, semi-circular flower beds B and D will be having equal diameters and hence equal areas.
So, Area of semi-circular flower bed D = 981.25
Total area of all the semi-circular flower beds is equal to the sum of all the areas of semi-circular beds A, B, C and D.
Total area of semi-circular flower beds = Area of semi-circular flower bed A + Area of semi-circular flower bed B + Area of semi-circular flower bed C + Area of semi-circular flower bed D
Since, Total cost of levelling the semi-circular flower beds = Rate of levelling the semi-circular flower beds
Therefore, the total cost of levelling the semi-circular flower beds at 60 paisa per square metre is Rs 5887.5
Note- In these types of problems, it is very important to make sure that if the unit of rate of levelling the semi-circular flower beds is Rs per
Complete step-by-step answer:
Given, Length of the rectangular park= 100 m
Breadth of the rectangular park= 50 m

Since, 1 paisa = Rs
Rate of levelling the semi-circular flower beds= 60 paisa per
The cost of levelling the semi-circular flower beds which are there around the rectangular park is required. This cost of levelling depends upon the total area of the semi-circular flower beds.
Clearly, from the figure we can see that there are a total four semi-circular flower beds around the rectangular park out of which two of them (A and C) have equal diameters and the other two (B and D) also have equal diameters. If diameters are equal that means radius is also equal and hence areas will also be equal.
From the figure, Diameter of semi-circular flower bed A = Length of rectangular park = 100 m
As we know that, Radius of a circle
So, Radius of semi-circular flower bed A
Also we know that, Area of a semi-circle with a radius R
Area of semi-circular flower bed A
Since, semi-circular flower beds A and C will be having equal diameters and hence equal areas.
So, Area of semi-circular flower bed C = 3925
Again from the figure, Diameter of semi-circular flower bed B = Breadth of rectangular park = 50 m
As we know that, Radius of a circle
So, Radius of semi-circular flower bed B
Also we know that, Area of a semi-circle with a radius R
Area of semi-circular flower bed B
Since, semi-circular flower beds B and D will be having equal diameters and hence equal areas.
So, Area of semi-circular flower bed D = 981.25
Total area of all the semi-circular flower beds is equal to the sum of all the areas of semi-circular beds A, B, C and D.
Total area of semi-circular flower beds = Area of semi-circular flower bed A + Area of semi-circular flower bed B + Area of semi-circular flower bed C + Area of semi-circular flower bed D
Since, Total cost of levelling the semi-circular flower beds = Rate of levelling the semi-circular flower beds
Therefore, the total cost of levelling the semi-circular flower beds at 60 paisa per square metre is Rs 5887.5
Note- In these types of problems, it is very important to make sure that if the unit of rate of levelling the semi-circular flower beds is Rs per
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

Whom did king Ashoka send to Sri Lanka to spread Buddhism class 7 social science CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

How many crores make 10 million class 7 maths CBSE

AIM To prepare stained temporary mount of onion peel class 7 biology CBSE

Find HCF and LCM of 120 and 144 by using Fundamental class 7 maths CBSE





