Question

A regular hexagonal pyramid whose base perimeter is 60 cm has an altitude of 30 cm, the volume of the pyramid (in cu.cm) is:
(a) 2958
(b) 2598
(c) 2859
(d) 2589

Answer 1

Hint: We are given the perimeter of a regular hexagonal box. We know that the perimeter of the hexagon is given by the formula 6a. From this, we will be able to find the side (a). Once we get the length of the side, we will find the area of the base. The base consists of 6 equilateral triangles, so the area of the base by the formula 6 x area of the equilateral triangle and at last we will find the volume using the formula, \[V=\dfrac{1}{3}\times \left( \text{Base Area} \right)\times \text{Height}\text{.}\]

Complete step-by-step answer:
We are given a regular hexagonal pyramid whose base perimeter is 60 cm and the height is 30 cm. We want to find its volume. We will first have to find the side of the base of the hexagonal pyramid.

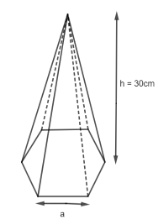
As we have the base of the regular hexagon, so each side has equal length and we will assume the side as a cm.
Now, we know that the perimeter of any figure is the length of its outline. So,
The Perimeter of the Hexagonal base = Sum of the length of all sides
The hexagon has 6 sides, so we get,
\[\text{Perimeter of the base}=a+a+a+a+a+a\]
And we have the perimeter of the base as 60cm, so we get,
\[\Rightarrow 60cm=6a\]
Dividing both the sides by 6, we get,
\[\Rightarrow a=\dfrac{60}{6}\]
\[\Rightarrow a=10cm\]
Now, we have to find the volume of the hexagonal pyramid.
We know that the volume of the hexagonal pyramid is given as
\[\text{Volume}=\dfrac{1}{3}\times \left( \text{Base Area} \right)\times \text{Height}\]
So, to find the volume, we need the base area of the hexagonal pyramid. The base of the hexagonal pyramid consists of 6 equilateral triangles with side 10 cm.

So,
\[\text{Area of Base}=6\times \text{Area of Equilateral Triangle}\]
We know that area of the equilateral triangle is given by the formula \[\dfrac{\sqrt{3}}{4}\times {{\left( \text{side} \right)}^{2}}.\]
As the side is 10cm, we get,
\[\text{Area of equilateral triangle}=\dfrac{\sqrt{3}}{4}\times {{\left( 10 \right)}^{2}}\]
\[\Rightarrow \text{Area of equilateral triangle}=\dfrac{\sqrt{3}}{4}\times 100\]
Simplifying further, we get,
\[\Rightarrow \text{Area of equilateral triangle}=25\sqrt{3}\]
Now, we have the area of the equilateral triangle. So,
\[\text{Area of Base}=6\times \text{Area of Equilateral Triangle}\]
So, we will put the values in the above formula, we will get,
\[\text{Area of Base}=6\times \text{2}\sqrt{3}\]
\[\Rightarrow \text{Area of Base}=150\sqrt{3}c{{m}^{2}}\]
Now, we have the area of the base as \[150\sqrt{3}c{{m}^{2}}\] and the height as 30 cm.
So,
\[\text{Volume}=\dfrac{1}{3}\times \left( \text{Base Area} \right)\times \text{Height}\]
We will get,
\[\Rightarrow \text{Volume}=\dfrac{1}{3}\times \left( \text{150}\sqrt{3} \right)\times \text{30}\]
\[\Rightarrow \text{Volume}=1500\sqrt{3}c{{m}^{3}}\]
Simplifying further, we get,
\[\Rightarrow \text{Volume}=2598\text{c}{{\text{m}}^{3}}\]
So, the correct answer is “Option B”.

Note: The alternate method is the volume of the hexagonal pyramid is given as
\[V=\dfrac{\sqrt{3}}{2}\times {{\left( a \right)}^{2}}\times h\]
where a is the side of the hexagon and h is the height.

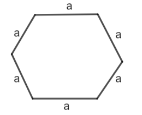
As we have the perimeter as 60cm, we can write
\[\text{Perimeter of the base}=a+a+a+a+a+a\]
\[\Rightarrow 60=6a\]
Solving for a, we get,
\[\Rightarrow a=10cm\]
We are already given that the height, h = 30cm. So, using a = 10 and h = 30 cm, we get,
\[\text{Volume}=\dfrac{\sqrt{3}}{2}\times {{a}^{2}}\times h\]
\[\Rightarrow \text{Volume}=\dfrac{\sqrt{3}}{2}\times {{10}^{2}}\times 30\]
Solving the terms, we get,
\[\Rightarrow \text{Volume}=1500\sqrt{3}c{{m}^{3}}\]
\[\Rightarrow \text{Volume}=2598c{{m}^{3}}\]