Question

A rhombus is symmetrical across ----.
A) its diagonals
B) its vertices
C) its sides
D) its angles

Answer 1

Hint: For any quadrilateral to be symmetric it should be divided into two equal parts. For a rhombus the sides and angles do not divide the figure in two equal parts and hence it cannot be symmetrical across either of them. Now we know that opposite angles of a rhombus are equal. We also know the diagonals bisect the opposite angles. Let us use these properties to determine if rhombus is symmetrical across its diagonals.

Complete step by step answer:
We will refer to the figure given below.

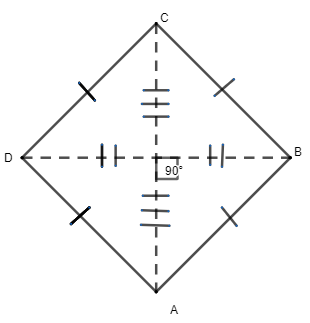
In the figure let us first consider the vertical diagonal AC.
Diagonal AC will divide the rhombus into two triangles, triangle ADC and triangle ABC.
Now, we will test these triangles for congruency.
In \[\Delta ADC\,and\,\Delta ABC\]
We know that opposite angles of a rhombus are equal.
\[\therefore \angle ADC = \angle ABC\]
Now, the diagonal AC divides the angles A and C in two equal parts.
Hence, we can say that
\[\angle DAC = \angle BAC\,\,and\,\angle DCA = \angle BCA\]
Therefore, by AAA -Test of congruence we can see that \[\,\Delta ADC\, and \,\Delta ABC\] are congruent.
Now, as the same properties apply to the other diagonal BD as well, we know that \[\Delta DCB\, and \,\Delta DAB\] will also be equal.
Hence the rhombus is symmetric across its diagonals.

So, the correct answer is “Option A”.

Note: First, we need to understand the definition of symmetric which is for any quadrilateral to be symmetric it should be divided into two equal parts Here we apply the properties of the rhombus. Now, when we check other options, we have vertices, sides and angles. From the figure, it is clear that the rhombus cannot be symmetric about its vertices or even angles, it is necessary to draw the diagonals from opposite vertices to make it symmetric. The sides also cannot act as lines of symmetry.