Question

A room has dimensions 5m \[ \times \]3m\[ \times \] 4m. The length of the longest pole which can be accommodated in the hall is
A. \[5\sqrt 2 \]m
B. 25m
C. 50m
D. \[2\sqrt 5 \]m

Answer 1

Hint: Use a rough figure for the dimensions of the room. Calculate the length of the longest pole that can be accommodated in the room by calculating the length of the cuboid formed with dimensions of room. Use formula of diagonal of cuboid. Bring all possible values out of square root and write the final answer.
* A cuboid having dimensions l, b and h will have a length of diagonal as \[\sqrt {{l^2} + {b^2} + {h^2}} \].

Complete answer:
We are given that room has dimensions 5m \[ \times \]3m\[ \times \] 4m
Then we can write the length of room i.e. \[l = 5\]
We can write breadth of the room i.e. \[b = 3\]
We can write height of the room i.e. \[h = 4\]
Draw a figure for room having dimensions given above

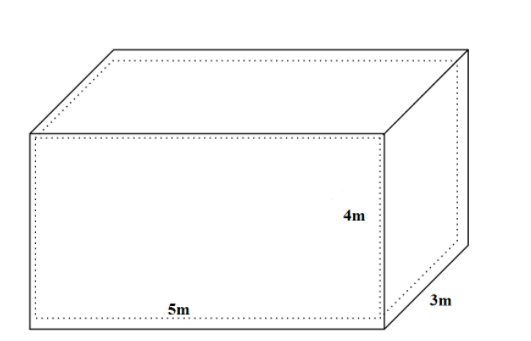
Now we can see that if a pole is taken in any direction of the room it will be either of length 5m, 4, or 3m.
Since we know the diagonal of a cuboid has the largest length then we calculate the length of the diagonal of the cuboidal room.
\[ \Rightarrow \]Length of diagonal \[ = \sqrt {{l^2} + {b^2} + {h^2}} \]
Substitute the value of \[l = 5,b = 3,h = 4\]in the formula
\[ \Rightarrow \]Length of diagonal \[ = \sqrt {{5^2} + {3^2} + {4^2}} \]
Calculate the squares of terms under the square root
\[ \Rightarrow \]Length of diagonal \[ = \sqrt {25 + 9 + 16} \]
Calculate the sum under the square root
\[ \Rightarrow \]Length of diagonal \[ = \sqrt {50} \]
\[ \Rightarrow \]Length of diagonal \[ = \sqrt {25 \times 2} \]
We know \[25 = {5^2}\]
\[ \Rightarrow \]Length of diagonal \[ = \sqrt {{5^2} \times 2} \]
\[ \Rightarrow \]Length of diagonal \[ = 5\sqrt 2 \]
\[\therefore \]The length of the longest pole which can be accommodated in the hall is \[5\sqrt 2 \]m

\[\therefore \]Option A is correct.

Note:
Many students make the mistake of writing the value of length of longest pole as the longest dimension given in the question i.e. 5m which is wrong, keep in mind in a cuboidal figure the length of diagonal is the longest.