Question

A simple pendulum is suspended from a peg on a vertical wall. The pendulum is pulled away from the wall to a horizontal position (see fig.) and released. The ball hits the wall, the coefficient of restitution being \[\dfrac{2}{{\sqrt 5 }}\]. What is the minimum number of collisions after which the amplitude of oscillation becomes less than 60 degrees?

Answer 1

Hint: Use the relation between the kinetic energy and potential energy of the simple pendulum at original and extreme position. Use the formula for the coefficient of restitution of the simple pendulum.

Formula used:
The kinetic energy \[K\] of an object is
\[K = \dfrac{1}{2}m{v^2}\] …… (1)
Here,\[m\] is the mass of the object and \[v\] is the velocity of the object.
The cosine of an angle \[\theta \] is
\[\cos \theta = \dfrac{{{\text{Adjacent side}}}}{{{\text{Hypotenuse}}}}\] …… (2)
The potential energy \[U\] of the object is
\[U = mgh\] …… (3)
Here, \[m\] is the mass of the object, \[g\] is the acceleration due to gravity and \[h\] is the height of the object from the ground.
The coefficient of restitution \[e\] for the simple pendulum is
\[e = \dfrac{v}{u}\] …… (4)
Here, \[u\] and \[v\] are the velocity of the pendulum before and after impact respectively.

Complete step by step answer:
The simple pendulum of mass \[m\] is suspended from a peg on a vertical wall. It is pulled away from the wall in the horizontal position and then released to hit the wall.
We have to calculate the number of collisions after which the amplitude of oscillation of the pendulum becomes less than \[60^\circ \].
Draw the diagram of the simple pendulum for the initial and after impact situation of the pendulum.

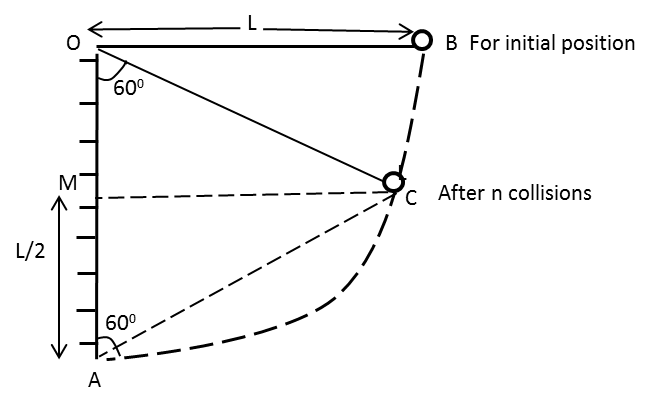
For the initial position of the pendulum, the height attained by the pendulum is \[L\].
Calculate the height AM attained by the bob of the pendulum after n collisions.
Suppose the amplitude of oscillations of the pendulum becomes \[60^\circ \] after a collision.
According to the law of triangles, the sum of all three angles of a triangle must be \[180^\circ \].
The triangle AOC in the figure is an equilateral triangle with each angle of \[180^\circ \].
\[\therefore \angle {\text{MAC}} = 60^\circ \]
Calculate the cosine of \[60^\circ \] to determine the height AM.
\[\cos 60^\circ = \dfrac{{{\text{AM}}}}{L}\]
\[ \Rightarrow \dfrac{1}{2} = \dfrac{{{\text{AM}}}}{L}\]
\[ \Rightarrow {\text{AM}} = \dfrac{L}{2}\]
Suppose the initial velocity of the pendulum is \[u\]. After every impact (collision), the velocity \[v\] decreases.
After n collisions, the velocity of the pendulum becomes
\[v = {e^n}u\]
Here, \[e\] is the coefficient of restitution.
At the original position, the pendulum bob has kinetic energy and at the extreme position, the total kinetic energy of the bob is converted into the potential energy.
Hence, for the first impact, kinetic energy \[{K_i}\] at original position is equal to potential energy \[{U_i}\] at horizontal position.
\[{K_i} = {U_i}\]
Substitute \[\dfrac{1}{2}m{u^2}\] for \[{K_i}\] and \[mgL\] for \[{U_i}\] in the above equation.
\[\dfrac{1}{2}m{u^2} = mgL\]
\[ \Rightarrow \dfrac{1}{2}{u^2} = gL\]
Rearrange the above equation for \[u\].
\[u = \sqrt {2gL} \]
After n collisions, kinetic energy \[{K_n}\] at original position is equal to potential energy \[{U_n}\] at decreased height.
\[{K_n} = {U_n}\]
Substitute \[\dfrac{1}{2}m{v^2}\] for \[{K_n}\] and \[mg\dfrac{L}{2}\] for \[{U_n}\] in the above equation.
\[\dfrac{1}{2}m{v^2} = mg\dfrac{L}{2}\]
\[ \Rightarrow {v^2} = gL\]
Rearrange the above equation for \[v\].
\[v = \sqrt {gL} \]
Now calculate the number of collisions after which the amplitude of oscillation becomes less than \[60^\circ \].
Rearrange equation (4) for the coefficient of restitution \[{e^n}\] after n collisions.
\[{e^n} = \dfrac{v}{u}\]
Substitute \[\sqrt {2gL} \] for \[u\], \[\sqrt {gL} \] for \[v\] and \[\dfrac{2}{{\sqrt 5 }}\] for \[e\] in equation (4).
\[{\left( {\dfrac{2}{{\sqrt 5 }}} \right)^n} = \dfrac{{\sqrt {gL} }}{{\sqrt {2gL} }}\]
\[ \Rightarrow {\left( {\dfrac{2}{{\sqrt 5 }}} \right)^n} = \dfrac{1}{{\sqrt 2 }}\]
Take a log on both sides of the above equation.
\[\log {\left( {\dfrac{2}{{\sqrt 5 }}} \right)^n} = {\text{log}}\dfrac{1}{{\sqrt 2 }}\]
\[ \Rightarrow n\log \left( {\dfrac{2}{{\sqrt 5 }}} \right) = {\text{log}}\dfrac{1}{{\sqrt 2 }}\]
\[ \Rightarrow n\left( {\log 2 - \log \sqrt 5 } \right) = \left( {\log 1 - \log \sqrt 2 } \right)\]
\[ \Rightarrow n\left( {0.6931 - 0.8047} \right) = \left( {0 - 0.3465} \right)\]
\[ \Rightarrow n = 3.104\]
\[ \Rightarrow n \approx 4\]
Hence, the number of collisions after which the amplitude of oscillation becomes less than 60 degree is 4.

Note:
Take logarithmic log and not the natural log of the equation while solving for n.
It is pulled away from the wall in the horizontal position and then released to hit the wall.
At the original position, the pendulum bob has kinetic energy and at the extreme position, the total kinetic energy of the bob is converted into the potential energy.