Question

A spherical body of diameter D is falling in viscous medium. Its terminal velocity is equal to:
 $\begin{align}
  & A){{V}_{1}}\propto {{D}^{\dfrac{1}{2}}} \\
 & B){{V}_{1}}\propto {{D}^{\dfrac{3}{2}}} \\
 & C){{V}_{1}}\propto {{D}^{2}} \\
 & D){{V}_{1}}\propto {{D}^{\dfrac{5}{2}}} \\
\end{align}$

Answer 1

Hint: Terminal velocity of a body is the constant value of speed that a freely falling body acquires, when the resistance of the medium through which it is falling prevents further acceleration of the body. In order to calculate the terminal velocity of a body in a liquid of density $\rho $, we need to apply Stoke’s law and balance the downward gravitational force of the body with upthrust and viscous force acting on that body.

Complete answer:
Terminal velocity of a body is observed when the sum of drag force and buoyant force becomes equal to the downward gravitational force that is acting on the body. The acceleration of the body is zero as the net force acting on the body is zero.
As the body falls through the medium, a stage reaches when the true weight of the body is just equal to the sum of the upward thrust due to buoyancy, that is, upthrust and the upward viscous drag. At this stage, there is no net force to accelerate the moving body. Hence it starts falling with a constant velocity called terminal velocity.
Now, let us consider a spherical body of radius r and density ${{\rho }_{0}}$ is falling through a medium of density $\rho $.

  

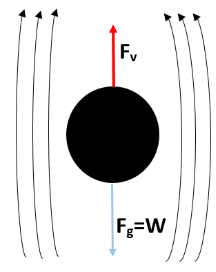
Now, true weight of the body will be given as,
$W=\text{Volume}\times \text{Density}\times \text{g}$
Volume of a spherical body is, $V=\dfrac{4}{3}\pi {{r}^{3}}$.
Where, r is the radius of the sphere.
Therefore,
$\begin{align}
  & W=\text{Volume}\times \text{Density}\times \text{g } \\
 & \Rightarrow W=\dfrac{4}{3}\pi {{r}^{3}}{{\rho }_{0}}g \\
\end{align}$
Now, let’s say that ${{F}_{T}}$ is the weight of the liquid displaced.
$\begin{align}
  & {{F}_{T}}=\text{Volume of liquid displaced}\times \text{Density}\times \text{g} \\
 & \Rightarrow {{F}_{T}}=\dfrac{4}{3}\pi {{r}^{3}}\rho g \\
\end{align}$
Now, if v is the terminal velocity of the body, then according to Stoke’s law, upward viscous drag is given by,
${{F}_{V}}=6\pi \eta rv$
Where, r is the radius of the sphere.
     And $\eta $ is the viscosity of the liquid.
So, when the spherical body acquires terminal velocity,
 ${{F}_{T}}+{{F}_{V}}=W$
Substituting the values,
${{F}_{T}}=\dfrac{4}{3}\pi {{r}^{3}}\rho g$
${{F}_{V}}=6\pi \eta rv$
$W=\dfrac{4}{3}\pi {{r}^{3}}{{\rho }_{0}}g$
We will get,
$\begin{align}
  & \Rightarrow \dfrac{4}{3}\pi {{r}^{3}}\rho g+6\pi \eta rv=\dfrac{4}{3}\pi {{r}^{3}}{{\rho }_{0}}g \\
 & \Rightarrow 6\pi \eta rv=\dfrac{4}{3}\pi {{r}^{3}}\left( {{\rho }_{0}}-\rho \right)g \\
 & \Rightarrow v=\dfrac{2{{r}^{2}}\left( {{\rho }_{0}}-\rho \right)g}{9\eta } \\
\end{align}$
So, the terminal velocity of a spherical body falling through a viscous medium is found to be,
$v=\dfrac{2{{r}^{2}}\left( {{\rho }_{0}}-\rho \right)g}{9\eta }$
From which, we can understand that,
$v\propto {{r}^{2}}$
Where, r is the radius of the sphere. But we know,
$r=\dfrac{D}{2}$
Where, D is the diameter of the sphere.
$\Rightarrow v\propto {{D}^{2}}$
So, we can conclude that terminal velocity of a spherical body is related to its diameter as $v\propto {{D}^{2}}$.

So, the correct answer is “Option C”.

Note:
While reaching the terminal velocity, the net force on a body will be zero and it will be having zero acceleration. We must also remember that terminal velocity of a spherical body is directly proportional to the square of its radius and inversely proportional to coefficient of viscosity of the medium. It also depends on the density of the body as well as the medium.