Question

A square and a regular hexagon have equal perimeters. Then their areas are in the ratio:

Answer 1

Hint: Here from the given perimeters we will find the side of square and hexagon. And with the help of it we will find the ratio of their areas.

Formula used:

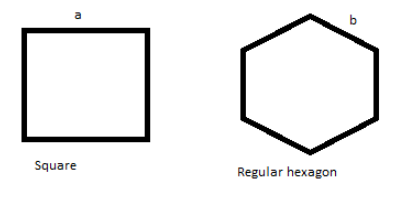
Let, the side of a square is a
The perimeter of a square is = 4a
The area of a square is \[{a^2}\]
Let, the side of a regular hexagon is b
The perimeter of the regular hexagon is = 6b
The area of a square is \[\dfrac{{3\sqrt 3 }}{2}{b^2}\]

Complete step-by-step answer:
It is given that the square and a regular hexagon have equal perimeters.
If the side of a square is a
The perimeter of the square is = 4a
And if the side of the regular hexagon is b,
The perimeter of the regular hexagon is = 6b
Then by the given condition, we have
\[4a = 6b\]
Let us solve the above equation to find “a”, we get,
\[a = \dfrac{{6b}}{4} = \dfrac{{3b}}{2}\]…..(1)
Let us mark it as equation (1)
The area of the square is \[{a^2}\]when the side of the square is a.
The area of the regular hexagon is \[\dfrac{{3\sqrt 3 }}{2}{b^2}\]when the side of the regular hexagon is b.
Then the ratio of the area of the square and the regular hexagon is found by dividing both the areas,
\[\dfrac{{{a^2}}}{{\dfrac{{3\sqrt 3 }}{2}{b^2}}}\]
Let us substitute equation (1) in the above equation we get,
\[ = \dfrac{{{{(\dfrac{{3b}}{2})}^2}}}{{\dfrac{{3\sqrt 3 }}{2}{b^2}}}\]
Let us now solve the above equation we get,
\[ = \dfrac{{9{b^2}}}{4} \times \dfrac{2}{{3\sqrt 3 {b^2}}}\]
We have found that,
\[\dfrac{{{a^2}}}{{\dfrac{{3\sqrt 3 }}{2}{b^2}}}\]\[ = \dfrac{{\sqrt 3 }}{2}\]

Hence the ratio of the area of the square and the regular hexagon is \[\sqrt 3 :2\] .

Note:Square:
In geometry, a square is a regular quadrilateral that has four equal sides and four equal angles.
Hexagon:
A hexagon is a six-sided polygon or 6-gon.The total of the internal angles of a hexagon is 720°.
Ratio is found by dividing the values for which we have to find the ratio.