
A thin concavo-convex lens has two surfaces of radii of curvature R and 2R. The material of the lens has a refractive index
A. Will depend on the direction from which light is incident on it
B. Will be the same, irrespective of the direction from which light is incident on it
C. Will be equal to
D. Will be equal to
Answer
500.7k+ views
Hint: As here we have to find the focal length of a thin concavo – convex lens whose radii of curvatures are given so it can be solved by using lens maker’s formula for thin lenses.
Formula used: Lens maker formula for thin – lenses is given by
Where f is the focal length of a thin lens,
Complete step by step answer:
We know that a thin concavo convex lens with light from left to right is given by the figure below
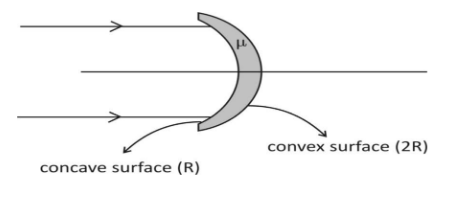
For light from left to right, the radii of curvature of first surface
The radii of curvature of second surface
Refractive index of material of lens
So, in this case, the focal length of the lens by lens maker formula is given by
When the light is incident from right to left in this concavo-convex lens, then as the given figure below we have
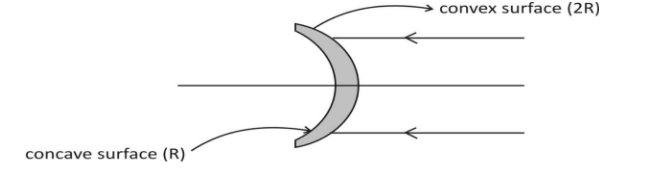
For light from right to left,
The radii of curvature of first surface
The radii of curvature of second surface
So, the focal length,
From (i) and (ii), it is clear that
Hence, option (D) is the correct option.
Note:: In case of incident light from left to right, then
It is negative because here the distances
When light is incident from right to left, then
Both are positive as both being measured in direction of incident light.
Formula used: Lens maker formula for thin – lenses is given by
Where f is the focal length of a thin lens,
Complete step by step answer:
We know that a thin concavo convex lens with light from left to right is given by the figure below

For light from left to right, the radii of curvature of first surface
The radii of curvature of second surface
Refractive index of material of lens
So, in this case, the focal length of the lens by lens maker formula is given by
When the light is incident from right to left in this concavo-convex lens, then as the given figure below we have

For light from right to left,
The radii of curvature of first surface
The radii of curvature of second surface
So, the focal length,
From (i) and (ii), it is clear that
Hence, option (D) is the correct option.
Note:: In case of incident light from left to right, then
It is negative because here the distances
When light is incident from right to left, then
Both are positive as both being measured in direction of incident light.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




