Question

A thin hollow prism of refracting angle 3 degrees filled with water gives a deviation of 1 degree. Find the refractive index of water.

Answer 1

Hint: In order to solve this problem we need to use the formula of angle of deviation related with the refractive index and the angle of prism. Drawing the figure will visualize the actual thing happening here. Doing this will solve your problem and will give you the right answer.

Complete answer:
It is said that a thin hollow prism of refracting angle 3 degrees filled with water gives a deviation of 1 degree. We need to find the refractive index of water.

As per the given condition we can draw the figure as:

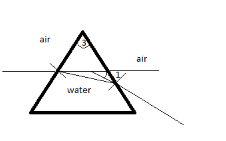
From the above figure we can clearly see that the incident ray is deviated by 1 degree.
So, we know the formula angle of deviation ($\delta $) = angle of prism (A)(1 – refractive index ($\mu $))
$ \Rightarrow \delta = A(1 - \mu )$
We need to find the refractive index of water here.
It is given that $\delta = 1$ and $A = 3$
On putting the values on above equation we get the value of equation as:
$
  1 = 3(1 - \mu ) \\
  \mu = 1 - \dfrac{1}{3} = \dfrac{2}{3} = 1.33 \\
$
So, the refractive index of water is 1.33.
Therefore the correct answer is 1.33.

Note:
In this problem you just need to know the formula $\delta = A(1 - \mu )$ and to also need to know that A ray of light is deflected twice in a prism. The sum of these deflections is the deviation angle. When the entrance and exit angles are equal, the deviation angle of a ray passing through a prism will be minimal. Knowing these things and drawing the diagram will give you the right answer. These types of problems of prism are very common and generally arise in most of the exams.