
A thin hollow prism of refracting angle
Answer
504.3k+ views
Hint: In a hollow prism, we can fill the cavity by adding any gas or other liquids. The refractive index of the fluid becomes the refractive index of the prism and we can apply the equations of the angle of deviation and refracting angle of the prism in this case. It should be kept in mind that the given prism is a thin hollow prism, or a small angled prism, and equations should be applied accordingly.
Formula used:
For refraction through a small angled prism:
Complete step by step solution:
An optical prism is defined as a transparent optical element with flat and polished surfaces that refract light. The two surfaces in a prism cannot be parallel to each other, one surface must be angled.
A hollow prism is a prism made using a glass plate with the prism’s centre part as empty. This cavity can be filled with gas or other liquids. Thus, a hollow prism is also called a fluid prism.
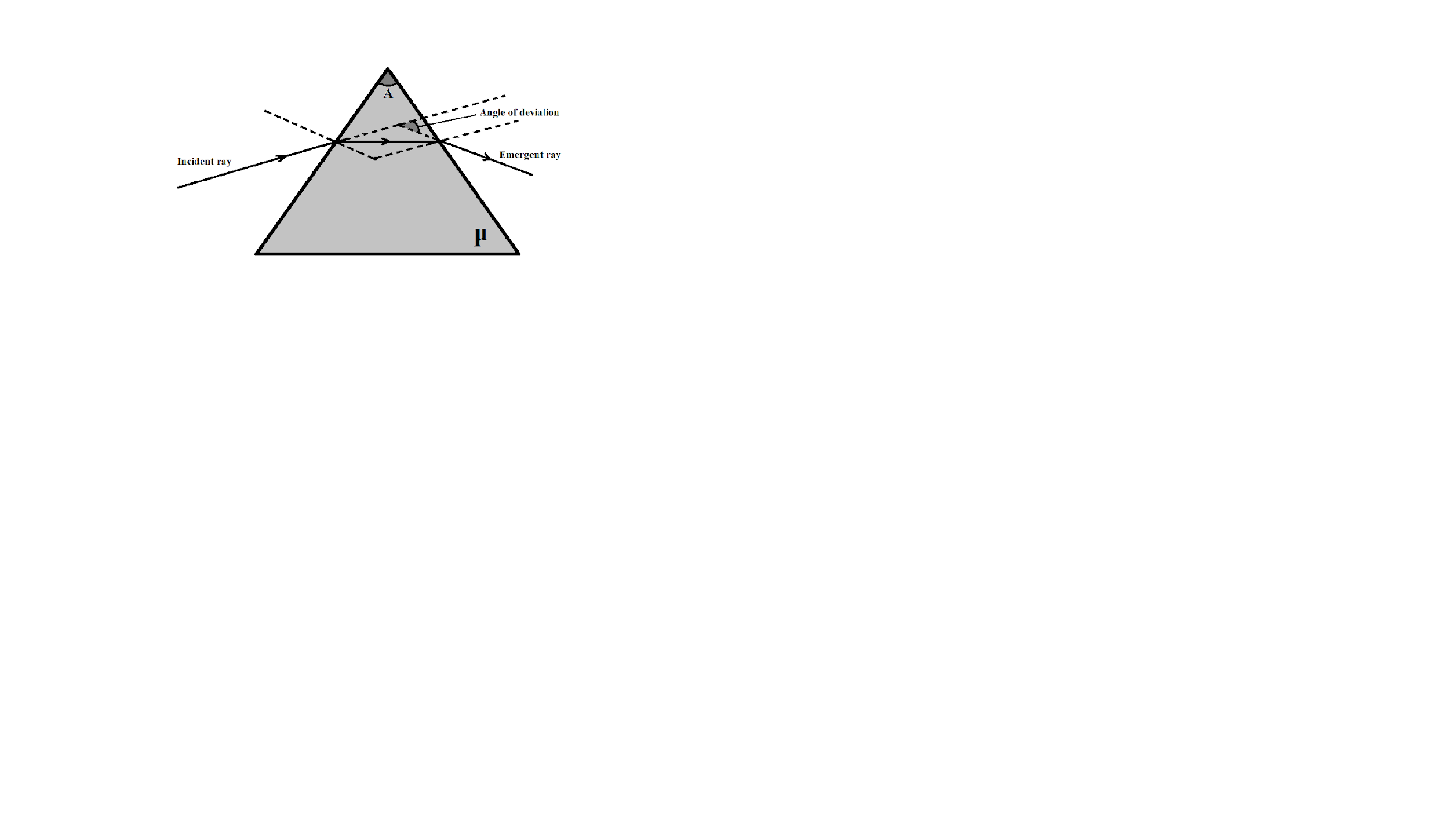
For refraction through a small angled prism, the angle of deviation is given by:
Where,
The angle of deviation in a small angled prism is directly proportional to the refracting angle of the prism. Also, the angle of deviation increases as the refractive index of the prism, or the fluid in case of hollow prism, increases.
We are given a thin hollow prism of refracting angle
We have,
We have to calculate the refractive index of water, say
For small angle of prism
Relation between deviation angle and angle of prism (refracting angle) is given as,
Or,
Putting values of
The refractive index of water is
Hence, the correct option is B.
Note:
In case of a hollow or fluid prism, the refractive index of prism is actually the refractive index of the fluid which is filled inside the prism. The above mentioned relation between angle of deviation and refracting angle of prism is valid only for the small angled prism, also called thin prism.
Formula used:
For refraction through a small angled prism:
Complete step by step solution:
An optical prism is defined as a transparent optical element with flat and polished surfaces that refract light. The two surfaces in a prism cannot be parallel to each other, one surface must be angled.
A hollow prism is a prism made using a glass plate with the prism’s centre part as empty. This cavity can be filled with gas or other liquids. Thus, a hollow prism is also called a fluid prism.

For refraction through a small angled prism, the angle of deviation is given by:
Where,
The angle of deviation in a small angled prism is directly proportional to the refracting angle of the prism. Also, the angle of deviation increases as the refractive index of the prism, or the fluid in case of hollow prism, increases.
We are given a thin hollow prism of refracting angle
We have,
We have to calculate the refractive index of water, say
For small angle of prism
Relation between deviation angle and angle of prism (refracting angle) is given as,
Or,
Putting values of
The refractive index of water is
Hence, the correct option is B.
Note:
In case of a hollow or fluid prism, the refractive index of prism is actually the refractive index of the fluid which is filled inside the prism. The above mentioned relation between angle of deviation and refracting angle of prism is valid only for the small angled prism, also called thin prism.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE




