
A thin uniform annular disc (see figure) of mass M has outer radius 4R and inner radius 3R. The work required to take a unit mass from point P on its axis to infinity is,


Answer
474.3k+ views
Hint: We must know that to bring a unit mass from point P to infinity, work has to be done against the gravitational field of the disc. So, we will find the gravitational potential of a small element of thickness
Complete step-by-step Solution:
Let us consider that the work needed to take a unit mass from point P to
Now, let us take such an element at distance r from the center of the disc as shown in the figure.
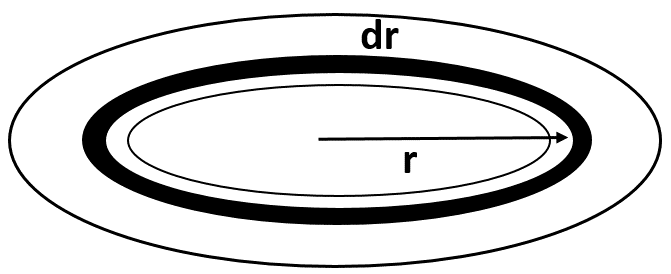
Now, the mass of the small element is given by,
So, the gravitational potential due to the entire disc is given by integrating the potential due to the number of these small elements within the limit 3R to 4R. That is,
Here, the distance of a small element from P is found using Pythagoras theorem.
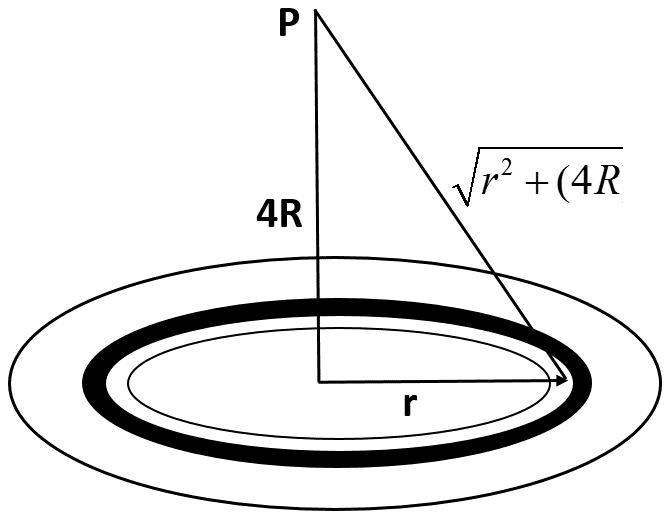
Now, substituting
Let us assume
Differentiating both sides, we will get
Now, when r=3R,
Now, when r=4R,
So, our limits will be changing according to this.
Or we can write this as,
Therefore the work required to take a unit mass from point P to infinity is found to be
So, the option B is correct.
Note:
The key element of this type of questions is understanding that the work needed to be done will be equal to the potential of the system. The negative sign will be there as work needed to be done against the potential. Also, the tricky part is changing the limits of the integration to our desired variable. So, we must be very careful while doing it.
Complete step-by-step Solution:
Let us consider that the work needed to take a unit mass from point P to
Now, let us take such an element at distance r from the center of the disc as shown in the figure.

Now, the mass of the small element is given by,
So, the gravitational potential due to the entire disc is given by integrating the potential due to the number of these small elements within the limit 3R to 4R. That is,
Here, the distance of a small element from P is found using Pythagoras theorem.

Now, substituting
Let us assume
Differentiating both sides, we will get
Now, when r=3R,
Now, when r=4R,
So, our limits will be changing according to this.
Or we can write this as,
Therefore the work required to take a unit mass from point P to infinity is found to be
So, the option B is correct.
Note:
The key element of this type of questions is understanding that the work needed to be done will be equal to the potential of the system. The negative sign will be there as work needed to be done against the potential. Also, the tricky part is changing the limits of the integration to our desired variable. So, we must be very careful while doing it.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




