
(a) What are coherent sources of light? State two conditions for two light sources to be coherent.
(b) Derive a mathematical expression for the width of the interference fringes obtained in Young’s double slit experiment with the help of a suitable diagram.
(c) If
Answer
391.5k+ views
Hint:Try to visualize the diagram and use different approximations to solve the question. General geometry can be used. The observations of Young’s double-slit experiment will give a perspective about the occurrence of interference. Coherency is the key to implement the experiment successfully.
Complete step by step answer:
(a) When two light sources produce waves having the same frequency and have a constant phase difference with time, they are called to be coherent. The two conditions for two light sources to be coherent are as follows:
-The coherent sources of light must have originated from a single source and must be monochromatic (that is they have a single wavelength) in nature.
-The path difference between the light waves from the two sources must be small.
(b) Let, a monochromatic source of light with wavelength
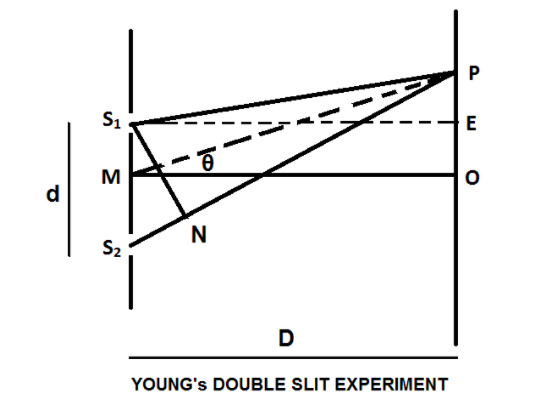
Now, the light waves from the slits
Let
Consider the right-angled triangles in the figure, we get:
Simplify the equation:
Approximate that,
So, Path difference
The condition for bright fringe at point P on the screen is:
The condition for dark fringe at point P on the screen is:
Where
In order to find the fringe width,
The fringe of
So, the fringe width,
(c) If
Where
Note: The fringe width for both the bright and dark fringes will be the same. This is because air is present between the slits and the screen and thus acting as a parallel film. Therefore, the light will not deviate from its original path and produce fringes of equal widths. If a Plano-convex lens is used to produce interference then circular fringes will occur as the air film will be of variable thickness.
Complete step by step answer:
(a) When two light sources produce waves having the same frequency and have a constant phase difference with time, they are called to be coherent. The two conditions for two light sources to be coherent are as follows:
-The coherent sources of light must have originated from a single source and must be monochromatic (that is they have a single wavelength) in nature.
-The path difference between the light waves from the two sources must be small.
(b) Let, a monochromatic source of light with wavelength

Now, the light waves from the slits
Let
Consider the right-angled triangles in the figure, we get:
Simplify the equation:
Approximate that,
So, Path difference
The condition for bright fringe at point P on the screen is:
The condition for dark fringe at point P on the screen is:
Where
In order to find the fringe width,
The fringe of
So, the fringe width,
(c) If
Where
Note: The fringe width for both the bright and dark fringes will be the same. This is because air is present between the slits and the screen and thus acting as a parallel film. Therefore, the light will not deviate from its original path and produce fringes of equal widths. If a Plano-convex lens is used to produce interference then circular fringes will occur as the air film will be of variable thickness.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




