
A wheel has three spokes and in a uniform magnetic field perpendicular to its plane, with the axis of rotation of the wheel parallel to the magnetic field. When the wheel rotates with a uniform angular velocity
A)
B)
C)
D)
Answer
440.1k+ views
Hint: Spoke is one of some number of rods radiating from the center of a wheel. Spoke are connecting rods between bicycle hub and the rim the purpose of the spoke is to transfer the load between the hub and rim, spokes are made of steel and that steel may be a stainless steel or high carbon steel.
Complete step-by-step solution:
Faraday's law of induction which predicts how a magnetic field interacts with electric field to induce an electromotive force this phenomenon is known as electrometric induction. Electromagnetic induction principle is used in transformers, generators and many other devices.
Faraday’s first laws of electromagnetic induction states that when a conductor is rotating in a magnetic field an emf is induced in a circuit.
Faraday's second of electromagnetic induction states that the rate of change of flux linkages is equal to the induced emf
Negative sign represents the direction of the induced current and will be in the opposite direction.
Emf induced in a rotating conductor in uniform magnetic field
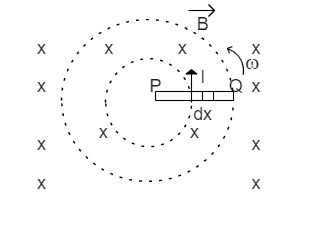
From the diagram you can see that uniform magnetic field
Motional emf induced in element
Total emf induced across P and Q is
The emf induced between the center and the rim will be
Induced emf
If the angular velocity is
So option B is the correct answer.
Note: Student the emf induced between the center and the rim can be obtained by faraday's laws and also by motional emf. Emf induced is independent upon the number of spokes. The number of spokes may be 3 or else 6 and it depends upon the velocity .Lighter wheel performances better than the stronger wheel.
Complete step-by-step solution:
Faraday's law of induction which predicts how a magnetic field interacts with electric field to induce an electromotive force this phenomenon is known as electrometric induction. Electromagnetic induction principle is used in transformers, generators and many other devices.
Faraday’s first laws of electromagnetic induction states that when a conductor is rotating in a magnetic field an emf is induced in a circuit.
Faraday's second of electromagnetic induction states that the rate of change of flux linkages is equal to the induced emf
Negative sign represents the direction of the induced current and will be in the opposite direction.
Emf induced in a rotating conductor in uniform magnetic field

From the diagram you can see that uniform magnetic field
Motional emf induced in element
Total emf induced across P and Q is
The emf induced between the center and the rim will be
Induced emf
If the angular velocity is
So option B is the correct answer.
Note: Student the emf induced between the center and the rim can be obtained by faraday's laws and also by motional emf. Emf induced is independent upon the number of spokes. The number of spokes may be 3 or else 6 and it depends upon the velocity .Lighter wheel performances better than the stronger wheel.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




