
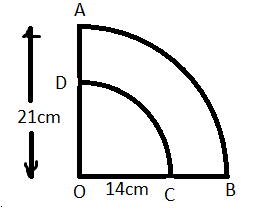
ABCD is a flower bed of OA = 21m and OC = 14m. Find the area of the bed. $\left( \text{take }\pi =\dfrac{22}{7} \right)$

Answer
504.9k+ views
Hint:For solving this problem,Assume given quadrant in the figure as a whole circle, then find the area of the circle having a larger diameter. Now, find the area of the circle having a smaller diameter. Subtract both the areas. It is evident from the figure that the required area is one fourth of the whole circle. In this way, we can obtain our answer.
Complete step-by-step answer:
According to the problem statement, we are given that ABCD is a flower bed having OA = 21m and OC = 14m. So, it can be shown diagrammatically as:
As we know that the area of a circle is $\pi {{r}^{2}}$, where r is the radius of the circle.
Assume given quadrant in the figure as whole circle then,
For larger circle having radius, OA = OB = 21m, the area would be
$\begin{align}
& \pi {{R}^{2}}=\pi {{\left( 21 \right)}^{2}} \\
& \Rightarrow \dfrac{22}{7}\times 21\times 21 \\
& \Rightarrow 22\times 3\times 21 \\
& \Rightarrow 1386{{m}^{2}} \\
\end{align}$
For smaller circle having radius, OC = 14m, the area would be
\[\begin{align}
& \pi {{r}^{2}}=\pi {{\left( 14 \right)}^{2}} \\
& \Rightarrow \dfrac{22}{7}\times 14\times 14 \\
& \Rightarrow 22\times 2\times 14 \\
& \Rightarrow 616{{m}^{2}} \\
\end{align}\]
Now, subtracting both the obtained areas, we get
$\begin{align}
& \pi {{R}^{2}}-\pi {{r}^{2}}=1386-616 \\
& \Rightarrow 770{{m}^{2}} \\
\end{align}$
This is the area of the complete circle but in the given figure, we are required only the area of the quadrant of a circle. Since the quadrant is one-fourth of the circle, the respective area will be one-fourth. Hence, required area of flower bed ABCD:
$\begin{align}
& A=\dfrac{1}{4}\times 770 \\
& A=192.5{{m}^{2}} \\
\end{align}$
Therefore, the obtained area of the flower bed ABCS is 192.5 square meter.
Note: This problem can alternatively be solved by using the formula of area of sector of a circle. The applicable formula can be stated as $\dfrac{\theta }{360}\times \left( \pi {{R}^{2}}-\pi {{r}^{2}} \right)$. By putting the values of both the radius and angle, we obtain the area of flower bed ABCD.
Complete step-by-step answer:
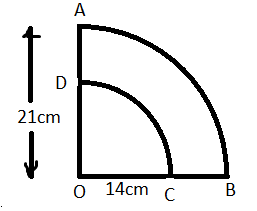
According to the problem statement, we are given that ABCD is a flower bed having OA = 21m and OC = 14m. So, it can be shown diagrammatically as:

As we know that the area of a circle is $\pi {{r}^{2}}$, where r is the radius of the circle.
Assume given quadrant in the figure as whole circle then,
For larger circle having radius, OA = OB = 21m, the area would be
$\begin{align}
& \pi {{R}^{2}}=\pi {{\left( 21 \right)}^{2}} \\
& \Rightarrow \dfrac{22}{7}\times 21\times 21 \\
& \Rightarrow 22\times 3\times 21 \\
& \Rightarrow 1386{{m}^{2}} \\
\end{align}$
For smaller circle having radius, OC = 14m, the area would be
\[\begin{align}
& \pi {{r}^{2}}=\pi {{\left( 14 \right)}^{2}} \\
& \Rightarrow \dfrac{22}{7}\times 14\times 14 \\
& \Rightarrow 22\times 2\times 14 \\
& \Rightarrow 616{{m}^{2}} \\
\end{align}\]
Now, subtracting both the obtained areas, we get
$\begin{align}
& \pi {{R}^{2}}-\pi {{r}^{2}}=1386-616 \\
& \Rightarrow 770{{m}^{2}} \\
\end{align}$
This is the area of the complete circle but in the given figure, we are required only the area of the quadrant of a circle. Since the quadrant is one-fourth of the circle, the respective area will be one-fourth. Hence, required area of flower bed ABCD:
$\begin{align}
& A=\dfrac{1}{4}\times 770 \\
& A=192.5{{m}^{2}} \\
\end{align}$
Therefore, the obtained area of the flower bed ABCS is 192.5 square meter.
Note: This problem can alternatively be solved by using the formula of area of sector of a circle. The applicable formula can be stated as $\dfrac{\theta }{360}\times \left( \pi {{R}^{2}}-\pi {{r}^{2}} \right)$. By putting the values of both the radius and angle, we obtain the area of flower bed ABCD.
Recently Updated Pages
Master Class 11 Accountancy: Engaging Questions & Answers for Success

Glucose when reduced with HI and red Phosphorus gives class 11 chemistry CBSE

The highest possible oxidation states of Uranium and class 11 chemistry CBSE

Find the value of x if the mode of the following data class 11 maths CBSE

Which of the following can be used in the Friedel Crafts class 11 chemistry CBSE

A sphere of mass 40 kg is attracted by a second sphere class 11 physics CBSE

Trending doubts
10 examples of friction in our daily life

Difference Between Prokaryotic Cells and Eukaryotic Cells

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

State and prove Bernoullis theorem class 11 physics CBSE

What organs are located on the left side of your body class 11 biology CBSE

Define least count of vernier callipers How do you class 11 physics CBSE




