
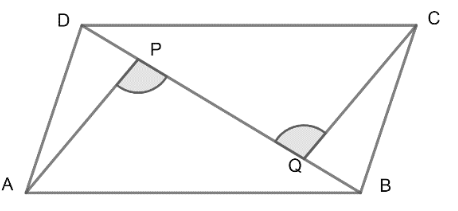
ABCD is a parallelogram AP and CQ are perpendicular drawn from vertices A and C on diagonal BD (see figure) show that AP = CQ.

Answer
512.1k+ views
3 likes
Hint: We will first start by using the fact that if two triangles are congruent then their corresponding parts are equal for proving two triangles congruent. We will use AAS congruency wherein we have to prove two angles and one side are equal.
Complete step-by-step solution -
Now, we have been given a parallelogram and AP and CQ are perpendicular drawn from vertices A and C and diagonal BD and we have to show that AP = CQ.
Now, we know that opposite sides of a parallelogram are parallel and equal. Therefore, we have,
Also, we have
Now, we know that the angle formed by a straight line is
Now, we have in
Hence,
Now, we know that by the corresponding part of the congruent triangle we have,
AP = CQ
Hence proved.
Note: It is important to note that we have used a fact that the sum of the linear pair of angles is
Complete step-by-step solution -
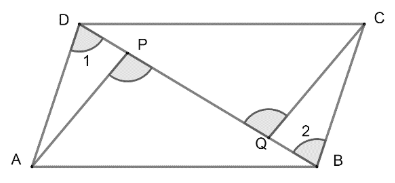
Now, we have been given a parallelogram and AP and CQ are perpendicular drawn from vertices A and C and diagonal BD and we have to show that AP = CQ.

Now, we know that opposite sides of a parallelogram are parallel and equal. Therefore, we have,
Also, we have
Now, we know that the angle formed by a straight line is
Now, we have in
Hence,
Now, we know that by the corresponding part of the congruent triangle we have,
AP = CQ
Hence proved.
Note: It is important to note that we have used a fact that the sum of the linear pair of angles is
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

What are the public facilities provided by the government? Also explain each facility

SI unit of electrical energy is A Joule B Kilowatt class 10 physics CBSE

The capital of British India was transferred from Calcutta class 10 social science CBSE

Identify the phrase s in the following sentence The class 10 english CBSE

How fast is 60 miles per hour in kilometres per ho class 10 maths CBSE




