
ABCD is a square, in which a circle is inscribed touching all the sides of the square. In the four corners of the square, 4 similar circles of equal radii are drawn, containing maximum possible area. What is the ratio of the area of the larger circle to that of the sum of the areas of four smaller circles?

Answer
498k+ views
Hint: Assume the area of the larger circle be ‘
Complete step-by-step answer:
Let the area of the larger circle be ‘
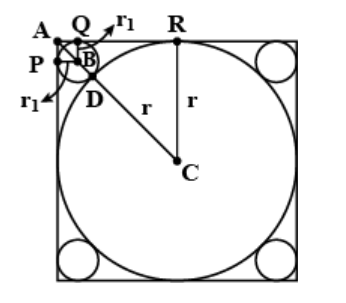
Now, see in triangle ACR,
CR = r = AR (radius of the larger circle)
Now, we can also write,
AC = CD + BD + AB
From the figure, we can say CD = r, DB =
To find AB, we need to apply Pythagora's theorem in triangle ABQ.
Therefore, in triangle ABQ,
AQ = BQ = r1 (radius of the smaller circles)
Therefore, AB =
Therefore, AB = r +
Applying Pythagoras theorem in triangle ACR,
Now, sum of areas of 4 smaller circles =
And, the area of the larger circle =
Therefore, the ratio of areas =
Using equation (1), we get the ratio of areas =
Hence, the answer is =
Note: Whenever these types of questions appear, assume the radius of larger circle as r and smaller circles as
Complete step-by-step answer:
Let the area of the larger circle be ‘

Now, see in triangle ACR,
CR = r = AR (radius of the larger circle)
Now, we can also write,
AC = CD + BD + AB
From the figure, we can say CD = r, DB =
To find AB, we need to apply Pythagora's theorem in triangle ABQ.
Therefore, in triangle ABQ,
AQ = BQ = r1 (radius of the smaller circles)
Therefore, AB =
Therefore, AB = r +
Applying Pythagoras theorem in triangle ACR,
Now, sum of areas of 4 smaller circles =
And, the area of the larger circle =
Therefore, the ratio of areas =
Using equation (1), we get the ratio of areas =
Hence, the answer is =
Note: Whenever these types of questions appear, assume the radius of larger circle as r and smaller circles as
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹31,500 per year
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility




