
AD bisects angle
Answer
508.8k+ views
Hint: At first we will try to find out the value of the angles of the triangle
Complete step-by-step answer:
It is given that,
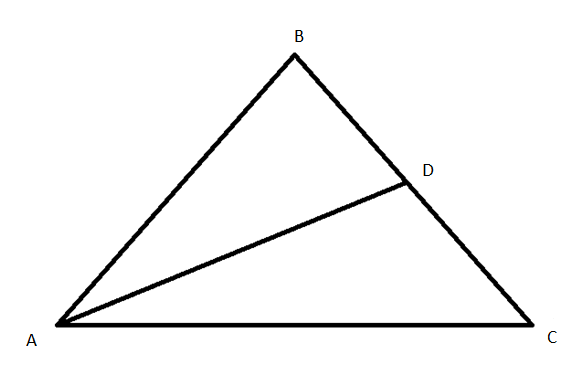
We know that sum of the all the angles of triangle is
Since,
We can write
So, now let us consider the triangle
Sum of all the angles is
That is the sum of the angles in the triangle
We know that
Similarly, from the triangle
Here we know that
It is also given that,
Let us multiply by
Now let us add
Also let us subtract
We know that from equation (1) and (2) we have
On substituting the values in the inequality we get,
Hence, we have shown that the angle
Note:
Let us consider,
Complete step-by-step answer:
It is given that,

We know that sum of the all the angles of triangle is
Since,
We can write
So, now let us consider the triangle
Sum of all the angles is
That is the sum of the angles in the triangle
We know that
Similarly, from the triangle
Here we know that
It is also given that,
Let us multiply by
Now let us add
Also let us subtract
We know that from equation (1) and (2) we have
On substituting the values in the inequality we get,
Hence, we have shown that the angle
Note:
Let us consider,
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

Whom did king Ashoka send to Sri Lanka to spread Buddhism class 7 social science CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

How many crores make 10 million class 7 maths CBSE

AIM To prepare stained temporary mount of onion peel class 7 biology CBSE

Find HCF and LCM of 120 and 144 by using Fundamental class 7 maths CBSE





