
Add the following algebraic expression using both horizontal and vertical methods.
Did you get the same answer with both methods?
$2{x^2} - 6x + 3; - 3{x^2} - x + 4;1 + 2x - 3{x^2}$
Answer
123k+ views
Hint: The horizontal method and the vertical method of adding the expression are two different ways to add the expression but the resultant answer is the same in both the cases.
Complete step-by-step answer:
Given algebraic expressions are $2{x^2} - 6x + 3; - 3{x^2} - x + 4;1 + 2x - 3{x^2}$
Assume the given algebraic expression as the functions:
$f\left( x \right) = 2{x^2} - 6x + 3$ ,
$g\left( x \right) = - 3{x^2} - x + 4$
$h\left( x \right) = 1 + 2x - 3{x^2}$
First, we add these expressions using the horizontal method. In the horizontal method of adding the expression, we first need to write all the expression in a horizontal line and then make groups of like terms, after that add all the like terms together.
$S\left( x \right) = f\left( x \right) + g\left( x \right) + h\left( x \right)$
Substitute $2{x^2} - 6x + 3$as the value of$f\left( x \right)$, $ - 3{x^2} - x + 4$as the value of$g\left( x \right)$and $1 + 2x - 3{x^2}$as the value of $h\left( x \right)$in the above expression:
$S\left( x \right) = \left( {2{x^2} - 6x + 3} \right) + \left( { - 3{x^2} - x + 4} \right) + \left( {1 + 2x - 3{x^2}} \right)$
Open the braces and express the terms:
$S\left( x \right) = 2{x^2} - 6x + 3 - 3{x^2} - x + 4 + 1 + 2x - 3{x^2}$
Now, collect all the like terms together and add them.
$S\left( x \right) = 2{x^2} - 3{x^2} - 3{x^2} - 6x - x + 2x + 3 + 4 + 1$
$S\left( x \right) = 4{x^2} - 5x + 8$
The sum of the given expression using the horizontal method is$4{x^2} - 5x + 8$.
Now, add the functions in the vertical method. In this method of adding expression, we first need to arrange the expressions in a vertical line in such a manner that the like terms and their signs are one below the other, and then add them.
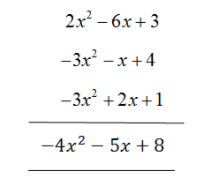
The sum of the given expression using the vertical method is$4{x^2} - 5x + 8$.
When we added the given expression using the horizontal method, then we have the solution:
$4{x^2} - 5x + 8$
And when we added the given expression using the vertical method, then we have the solution:
$4{x^2} - 5x + 8$
It can be seen that both solutions are the same. The conclusion is that we get the same answer using horizontal and vertical methods both.
Note: The like terms are the terms of the expression that have the same variables raised to the same power. In the given expressions, $2{x^2} - 6x + 3; - 3{x^2} - x + 4;1 + 2x - 3{x^2}$, the like terms are $\left( {2{x^2}, - 3{x^2}, - 3{x^2}} \right),\left( { - 6x, - x,2x} \right){\text{ and }}\left( {3,4,1} \right)$.
Complete step-by-step answer:
Given algebraic expressions are $2{x^2} - 6x + 3; - 3{x^2} - x + 4;1 + 2x - 3{x^2}$
Assume the given algebraic expression as the functions:
$f\left( x \right) = 2{x^2} - 6x + 3$ ,
$g\left( x \right) = - 3{x^2} - x + 4$
$h\left( x \right) = 1 + 2x - 3{x^2}$
First, we add these expressions using the horizontal method. In the horizontal method of adding the expression, we first need to write all the expression in a horizontal line and then make groups of like terms, after that add all the like terms together.
$S\left( x \right) = f\left( x \right) + g\left( x \right) + h\left( x \right)$
Substitute $2{x^2} - 6x + 3$as the value of$f\left( x \right)$, $ - 3{x^2} - x + 4$as the value of$g\left( x \right)$and $1 + 2x - 3{x^2}$as the value of $h\left( x \right)$in the above expression:
$S\left( x \right) = \left( {2{x^2} - 6x + 3} \right) + \left( { - 3{x^2} - x + 4} \right) + \left( {1 + 2x - 3{x^2}} \right)$
Open the braces and express the terms:
$S\left( x \right) = 2{x^2} - 6x + 3 - 3{x^2} - x + 4 + 1 + 2x - 3{x^2}$
Now, collect all the like terms together and add them.
$S\left( x \right) = 2{x^2} - 3{x^2} - 3{x^2} - 6x - x + 2x + 3 + 4 + 1$
$S\left( x \right) = 4{x^2} - 5x + 8$
The sum of the given expression using the horizontal method is$4{x^2} - 5x + 8$.
Now, add the functions in the vertical method. In this method of adding expression, we first need to arrange the expressions in a vertical line in such a manner that the like terms and their signs are one below the other, and then add them.
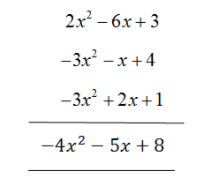
The sum of the given expression using the vertical method is$4{x^2} - 5x + 8$.
When we added the given expression using the horizontal method, then we have the solution:
$4{x^2} - 5x + 8$
And when we added the given expression using the vertical method, then we have the solution:
$4{x^2} - 5x + 8$
It can be seen that both solutions are the same. The conclusion is that we get the same answer using horizontal and vertical methods both.
Note: The like terms are the terms of the expression that have the same variables raised to the same power. In the given expressions, $2{x^2} - 6x + 3; - 3{x^2} - x + 4;1 + 2x - 3{x^2}$, the like terms are $\left( {2{x^2}, - 3{x^2}, - 3{x^2}} \right),\left( { - 6x, - x,2x} \right){\text{ and }}\left( {3,4,1} \right)$.
Recently Updated Pages
Difference Between Mutually Exclusive and Independent Events

Difference Between Area and Volume

Area of a Rhombus Formula | Perimeter and Area of Rhombus

Difference Between Power and Exponent: JEE Main 2024

JEE Main Exam Events 2025: Key Dates, Schedule, and Updates

NIT Andhra Pradesh Cutoff 2025: Check JEE Main Round Wise Cutoff Ranks

Trending doubts
JEE Mains 2025: Check Important Dates, Syllabus, Exam Pattern, Fee and Updates

JEE Main 2025 22 Jan Shift 1 Question Paper with Solutions

JEE Main Physics Question Paper with Answer Keys and Solutions

JEE Main Question Papers 2025

JEE Main 27 January 2024 Shift 1 Question Paper with Solutions

JEE Main Sample Paper (Set 1) with Solutions (2024-25)

Other Pages
NCERT Solutions for Class 9 Maths Chapter 9 Circles

NCERT Solutions for Class 9 Maths Chapter 11 Surface Area and Volume

NCERT Solutions for Class 9 Maths Chapter 11 Surface Areas And Volumes Ex 11.3

NCERT Solutions for Class 9 Maths Chapter 12 Statistics

NCERT Solutions for Class 9 Maths Chapter 10 Heron'S Formula

NCERT Solutions for Class 9 Maths In Hindi Chapter 1 Number System




