
Altitudes of a triangle are concurrent – prove by vector method.
Answer
436.4k+ views
Hint: To solve the question, we have to represent the information of sides and altitude of a triangle in vector form using basic principles of vectors. To prove that altitudes of a triangle are concurrent, we have to prove that the line segment joining the orthocentre and a vertex considering the altitudes drawn from the other two vertices of triangle meet at the orthocentre. To solve further, we have to apply the properties of the dot product to prove that the line segment joining the orthocentre and a vertex is perpendicular to the side of the triangle.
Complete step by step answer:
Let the given triangle be
Let AD, BE be the altitudes of the given triangle.
Let O be the orthocentre of the given triangle of position vectors at origin.
We know that orthocentre is the point of intersection of the altitudes. Thus, O is the point of intersection of AD and BE.
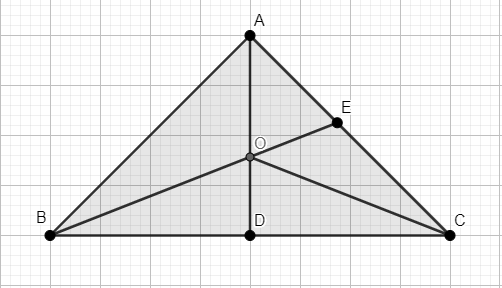
The vector representation of OA =
The vector representation of OB =
The vector representation of OC =
We know that AD is perpendicular to BC, by the definition of an altitude of a triangle.
Thus, we get that OA is perpendicular to BC.
The vector representation of the above statement is
Since we know that the dot product of perpendicular vectors is 0.
By solving the above equation, we get
We know that BE is perpendicular to CA, by the definition of an altitude of a triangle.
Thus, we get that OB is perpendicular to CA.
The vector representation of the above statement is
By adding the above equation and equation (1), we get
Thus, we get OC is perpendicular to BA.
This implies OC is an altitude of the given triangle.
Thus, the altitudes of a triangle are concurrent.
Note: The possibility of mistake can be, not analysing that proving altitudes of a triangle are concurrent is equal to proving that the line segment joining the orthocentre and a vertex considering the altitudes drawn from the other two vertices of triangle meet at the orthocentre. The possibility of mistake can be not applying the properties of the dot product to prove that the line segment joining the orthocentre and a vertex is perpendicular to the side of the triangle.
Complete step by step answer:
Let the given triangle be
Let AD, BE be the altitudes of the given triangle.
Let O be the orthocentre of the given triangle of position vectors at origin.
We know that orthocentre is the point of intersection of the altitudes. Thus, O is the point of intersection of AD and BE.

The vector representation of OA =
The vector representation of OB =
The vector representation of OC =
We know that AD is perpendicular to BC, by the definition of an altitude of a triangle.
Thus, we get that OA is perpendicular to BC.
The vector representation of the above statement is
Since we know that the dot product of perpendicular vectors is 0.
By solving the above equation, we get
We know that BE is perpendicular to CA, by the definition of an altitude of a triangle.
Thus, we get that OB is perpendicular to CA.
The vector representation of the above statement is
By adding the above equation and equation (1), we get
Thus, we get OC is perpendicular to BA.
This implies OC is an altitude of the given triangle.
Thus, the altitudes of a triangle are concurrent.
Note: The possibility of mistake can be, not analysing that proving altitudes of a triangle are concurrent is equal to proving that the line segment joining the orthocentre and a vertex considering the altitudes drawn from the other two vertices of triangle meet at the orthocentre. The possibility of mistake can be not applying the properties of the dot product to prove that the line segment joining the orthocentre and a vertex is perpendicular to the side of the triangle.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




