
An equi concave diverging lens of focal length
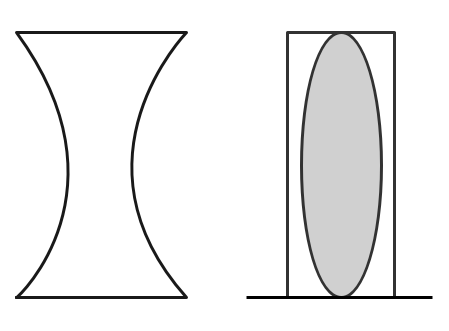

Answer
495.3k+ views
Hint: We know that the lens makers formula for thin lenses, where
Formula used:
Complete step by step answer:
We also know that the focal length
Now consider the focal length of the combination of two halves of the concave lens and the liquid with refractive index
Then clearly
Similarly,
But since the
We can write it as
An equiconvex lens of focal length
Then we can get,
Given
Thus the refractive index of the lens
Hence the answer is
Note:
The lens makers formula as the name suggests is used in the making of the lens. Also clearly, more the refractive index of the lens or combination of lens and liquid, less the focal length. Whereas, the more the radius of curvature of the spherical lens, the more is the focal length of the lens.
Formula used:
Complete step by step answer:
We also know that the focal length
Now consider the focal length of the combination of two halves of the concave lens and the liquid with refractive index
Then clearly
Similarly,
But since the
We can write it as
An equiconvex lens of focal length
Then we can get,
Given
Thus the refractive index of the lens
Hence the answer is
Note:
The lens makers formula as the name suggests is used in the making of the lens. Also clearly, more the refractive index of the lens or combination of lens and liquid, less the focal length. Whereas, the more the radius of curvature of the spherical lens, the more is the focal length of the lens.
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
What is the difference between resemblance and sem class 12 social science CBSE

Draw ray diagrams each showing i myopic eye and ii class 12 physics CBSE

Why do the transition elements have higher enthalpies class 12 chemistry CBSE

What are the advantages of parallel combination over class 12 physics CBSE

What are the causes of overloading class 12 physics CBSE

There is hypertension due to smoking Give scientific class 12 biology CBSE




