
An ideal flow of any fluid must fulfill the following
A. Newton’s law of viscosity
B. Newton’s law of motion
C. Pascal’s law
D. Continuity equation
Answer
475.8k+ views
Hint: For any type of flow of liquids, the “Continuity equation” must be satisfied. This is to satisfy the conservation of mass for the flow of liquid.
Complete step by step answer:
An ideal flow of any fluid must fulfill the “Continuity equation”. The continuity equation applies to all fluids whether incompressible (ideal) or compressible (non-ideal), Newtonian or non-Newtonian fluids. Continuity equation expresses the law of conservation of mass at each point in a fluid and therefore must be satisfied at every point in the field of flow.
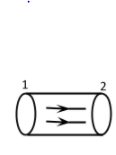
Let us consider a pipe of non-uniform length. The area at point 1 is greater than that of point 2. If mass ${m_1}$ of liquid is entering from point 1 and mass ${m_2}$of liquid is going out from point 2 in the field of flow, then by the law of conservation of mass, ${m_1}$must be equal to ${m_2}$.
So, ${m_1} = {m_2}\,...............\left( i \right)$
Let density of the liquid is $\rho $ and volume flowed from point 1 to point 2 in the field of flow are ${V_1}$and ${V_2}$respectively, then from equation (i).
${m_1} = {m_2}$
or $\rho {V_1} = \rho {V_2}$
or ${V_1} = {V_2}........\left( {ii} \right)$
Now dividing both side of equation (ii) by time `t’ , we have
$\dfrac{{{V_1}}}{t} = \dfrac{{{V_2}}}{t}.........\left( {iii} \right)$
The quantity $\dfrac{{volume}}{{time}}$in the field of flow represents volume flow rate (R) by which the liquid flows.
So, from equation (iii), we have
$\dfrac{{{V_1}}}{t} = \dfrac{{{V_2}}}{t}$
Or ${R_1} = {R_2}$
If the liquid enters into a pipe with rate ${R_1}$and goes out with rate ${R_2}$, then ${R_1} = {R_2}$.
So, the correct answer is “Option D”.
Note:
The rate of flow of liquid at any two points into the flow field must be the same. We can also say that when a fluid is in motion , its motion is in accordance with conservation of mass, that is mass rate of flow is the same across the length of flow of liquid which is nothing but an equation of continuity.
Complete step by step answer:
An ideal flow of any fluid must fulfill the “Continuity equation”. The continuity equation applies to all fluids whether incompressible (ideal) or compressible (non-ideal), Newtonian or non-Newtonian fluids. Continuity equation expresses the law of conservation of mass at each point in a fluid and therefore must be satisfied at every point in the field of flow.

Let us consider a pipe of non-uniform length. The area at point 1 is greater than that of point 2. If mass ${m_1}$ of liquid is entering from point 1 and mass ${m_2}$of liquid is going out from point 2 in the field of flow, then by the law of conservation of mass, ${m_1}$must be equal to ${m_2}$.
So, ${m_1} = {m_2}\,...............\left( i \right)$
Let density of the liquid is $\rho $ and volume flowed from point 1 to point 2 in the field of flow are ${V_1}$and ${V_2}$respectively, then from equation (i).
${m_1} = {m_2}$
or $\rho {V_1} = \rho {V_2}$
or ${V_1} = {V_2}........\left( {ii} \right)$
Now dividing both side of equation (ii) by time `t’ , we have
$\dfrac{{{V_1}}}{t} = \dfrac{{{V_2}}}{t}.........\left( {iii} \right)$
The quantity $\dfrac{{volume}}{{time}}$in the field of flow represents volume flow rate (R) by which the liquid flows.
So, from equation (iii), we have
$\dfrac{{{V_1}}}{t} = \dfrac{{{V_2}}}{t}$
Or ${R_1} = {R_2}$
If the liquid enters into a pipe with rate ${R_1}$and goes out with rate ${R_2}$, then ${R_1} = {R_2}$.

So, the correct answer is “Option D”.
Note:
The rate of flow of liquid at any two points into the flow field must be the same. We can also say that when a fluid is in motion , its motion is in accordance with conservation of mass, that is mass rate of flow is the same across the length of flow of liquid which is nothing but an equation of continuity.
Recently Updated Pages
Master Class 11 Accountancy: Engaging Questions & Answers for Success

Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Trending doubts
10 examples of friction in our daily life

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

State and prove Bernoullis theorem class 11 physics CBSE

What organs are located on the left side of your body class 11 biology CBSE

Write down 5 differences between Ntype and Ptype s class 11 physics CBSE




