
An infinite wire is bent in the form of L and carries a current
A) Zero
B)
C)
D)

Answer
501.9k+ views
Hint: The infinitely long wire bent in the shape of L constitutes two semi-infinite wires. The magnetic field at point O due to the two wires will be opposite in direction at the point of intersection as the current flow has different directions in the two wires.
Formula used:
The magnetic field at a point O for a finite wire carrying a current
Complete step by step answer:
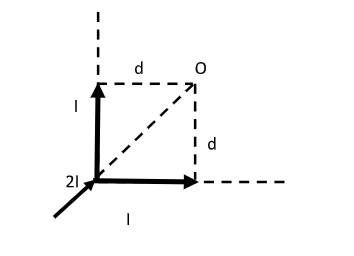
Step 1: Sketch a figure representing the infinitely long wire bent in the form of L and list the parameters involved.
Here, the infinitely long wire is bent in the shape of L. We can now treat this infinitely long wire as two semi-infinite wires. For each wire, one end is finite and the other end is infinitely long.
A current
A point O is considered such that the perpendicular distance between point O and the wire is
The magnetic field at point O is unknown.
Step 2: Find the magnetic field at point O due to the current in the wire placed along the vertical direction.
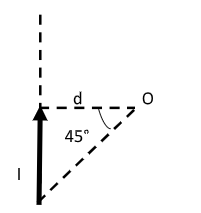
Let
We have the magnetic field at point O as
Here,
Also,
For the infinite end,
Then equation (1) becomes,
Therefore, the magnetic field at O due to the current in the wire placed along the vertical direction is
Step 3: Find the magnetic field at point O due to the current in the wire placed along the horizontal direction.
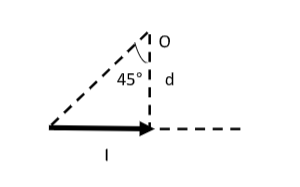
Let
We have the magnetic field at point O as
Here,
Also,
For the infinite end,
Then equation (2) becomes,
Therefore, the magnetic field at O due to the current in the wire placed along the horizontal direction is
Step 4: Find the net magnetic field at point O.
We thus have, the magnetic fields at point O due to the current in the two semi-infinite wires as given by equations (A) and (B) as
When the right-hand thumb rule is used at the point of intersection, the magnetic field lines will have two directions since the current flow along the two wires is different. The point O is essentially an imaginary point that actually exists at the point of intersection.
So, the net magnetic field at the point of intersection is
Therefore, the magnetic field at point O is zero.
Note:
The right-hand thumb rule gives us the direction of the magnetic field at a point when the direction of the current is known. According to the right-hand thumb rule we consider a current-carrying wire in our right hand. Now the thumb points in the direction of the current and all the finger curling around the wire will give the direction of the magnetic field.
Formula used:
The magnetic field at a point O for a finite wire carrying a current
Complete step by step answer:
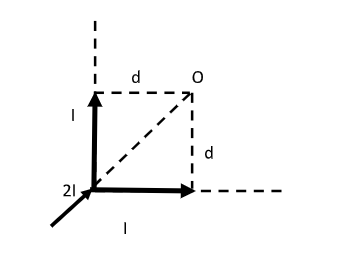
Step 1: Sketch a figure representing the infinitely long wire bent in the form of L and list the parameters involved.

Here, the infinitely long wire is bent in the shape of L. We can now treat this infinitely long wire as two semi-infinite wires. For each wire, one end is finite and the other end is infinitely long.
A current
A point O is considered such that the perpendicular distance between point O and the wire is
The magnetic field at point O is unknown.
Step 2: Find the magnetic field at point O due to the current in the wire placed along the vertical direction.

Let
We have the magnetic field at point O as
Here,
Also,
For the infinite end,
Then equation (1) becomes,
Therefore, the magnetic field at O due to the current in the wire placed along the vertical direction is
Step 3: Find the magnetic field at point O due to the current in the wire placed along the horizontal direction.

Let
We have the magnetic field at point O as
Here,
Also,
For the infinite end,
Then equation (2) becomes,
Therefore, the magnetic field at O due to the current in the wire placed along the horizontal direction is
Step 4: Find the net magnetic field at point O.
We thus have, the magnetic fields at point O due to the current in the two semi-infinite wires as given by equations (A) and (B) as
When the right-hand thumb rule is used at the point of intersection, the magnetic field lines will have two directions since the current flow along the two wires is different. The point O is essentially an imaginary point that actually exists at the point of intersection.
So, the net magnetic field at the point of intersection is
Therefore, the magnetic field at point O is zero.
Note:
The right-hand thumb rule gives us the direction of the magnetic field at a point when the direction of the current is known. According to the right-hand thumb rule we consider a current-carrying wire in our right hand. Now the thumb points in the direction of the current and all the finger curling around the wire will give the direction of the magnetic field.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




