
An isosceles triangle has perimeter 30 cm and each of the equal sides is 12 cm. Find the area of the triangle.
Answer
430.6k+ views
Hint: We have given the length of two sides as 12 cm so let us assume that the third side be x then take the sum of two sides of length 12 cm and the third side x and equate it to 30. From this we will get the third side. To find the area of the triangle, use the Heron’s formula which is equal to
Complete step-by-step answer:
In the below diagram, we have shown an isosceles triangle ABC with AB = AC = 12 cm.
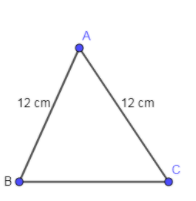
Let us assume that the length of the side BC is equal to x. We know that the perimeter of a triangle is the sum of all the sides of the triangle.
Perimeter of the triangle is equal to addition of AB, BC and CA. Adding all the three sides of the triangle we get,
It is given that the perimeter of the triangle is equal to 30 cm so equating the above expression we get,
From the above, we have got the length of the third side as 6 cm.
We are asked to find the area of the triangle. For that we are going to use the Heron’s formula which states that:
We are denoting the area of the triangle as
In the above formula, “s” represents the semi – perimeter of the triangle, “a, b and c” represents the sides of the triangle ABC.
Perimeter of the triangle is given as 30 cm so the semi perimeter is equal to half of the perimeter i.e. 15 cm.
We are taking
Hence, the area of the triangle is equal to
Note: The most common mistake that could happen in this problem is the calculation mistake. There is a trick to remember this Heron’s area formula.
The trick is that remembering root over is easy but then you might forget what is inside the root over which you can remember like first all no side is subtracting from the semi perimeter
Complete step-by-step answer:
In the below diagram, we have shown an isosceles triangle ABC with AB = AC = 12 cm.

Let us assume that the length of the side BC is equal to x. We know that the perimeter of a triangle is the sum of all the sides of the triangle.
Perimeter of the triangle is equal to addition of AB, BC and CA. Adding all the three sides of the triangle we get,
It is given that the perimeter of the triangle is equal to 30 cm so equating the above expression we get,
From the above, we have got the length of the third side as 6 cm.
We are asked to find the area of the triangle. For that we are going to use the Heron’s formula which states that:
We are denoting the area of the triangle as
In the above formula, “s” represents the semi – perimeter of the triangle, “a, b and c” represents the sides of the triangle ABC.
Perimeter of the triangle is given as 30 cm so the semi perimeter is equal to half of the perimeter i.e. 15 cm.
We are taking
Hence, the area of the triangle is equal to
Note: The most common mistake that could happen in this problem is the calculation mistake. There is a trick to remember this Heron’s area formula.
The trick is that remembering root over is easy but then you might forget what is inside the root over which you can remember like first all no side is subtracting from the semi perimeter
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




