
An object 5.0cm in length is placed at a distance of 20cm in front of a convex mirror of radius of curvature 30cm. Find the position of the image, its nature, and its size.
Answer
142.8k+ views
Hint: The convex and concave mirror are the two sides of a circular portion of a sphere. If you consider a circular portion of a sphere, applying the reflective coating on the outer side will make it a concave mirror and applying the reflective coating on the inner side will make it a convex mirror.
Irrespective of concave or convex mirror, the image formed will follow the mirror equation,
where, f = focal length of the mirror, v = distance of image from pole, u = distance of object from pole.
Complete step by step solution:
Consider the object placed at a distance of 20cm in front of a convex mirror of radius of curvature, R = 30cm.
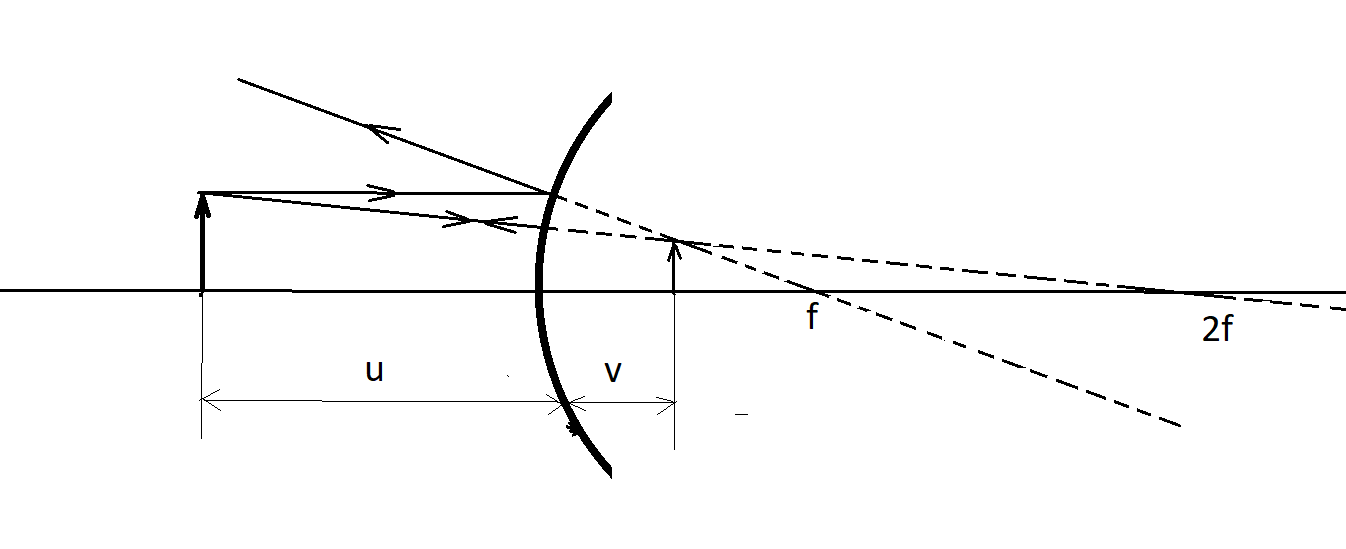 Distance of the object, u = – 20 cm
Distance of the object, u = – 20 cm
Focal length,
There are two rays emanating from the object,
i) First ray passes parallel to the principal axis and after deflection, when traced back, passes through primary focus, f.
ii) Second ray passes along the direction of secondary focus, 2f and retraces the same path as shown.
The image is formed at distance +v from the pole.
Applying the mirror equation, we get –
The image is formed at a distance of 8.57 metres from the convex mirror.
Magnification is defined as the ratio between the height of the object to the height of the image. It represents the change in the height of the object after the reflection.
The formula for magnification in a mirror is –
Since, the magnification is positive, the image is virtual and since it is lesser than one, it is diminished.
Therefore, The image is formed at a distance of 8.57 m from the pole and it is virtual and diminished.
Note: The students should exercise extreme caution while substituting the values of u and v in the equations. As you can see, in the above steps, the value of u is substituted with entire brackets first, and then, solved by removing the brackets. This is the best practice to avoid errors due to sign convention in these problems.
Irrespective of concave or convex mirror, the image formed will follow the mirror equation,
where, f = focal length of the mirror, v = distance of image from pole, u = distance of object from pole.
Complete step by step solution:
Consider the object placed at a distance of 20cm in front of a convex mirror of radius of curvature, R = 30cm.
 Distance of the object, u = – 20 cm
Distance of the object, u = – 20 cmFocal length,
There are two rays emanating from the object,
i) First ray passes parallel to the principal axis and after deflection, when traced back, passes through primary focus, f.
ii) Second ray passes along the direction of secondary focus, 2f and retraces the same path as shown.
The image is formed at distance +v from the pole.
Applying the mirror equation, we get –
The image is formed at a distance of 8.57 metres from the convex mirror.
Magnification is defined as the ratio between the height of the object to the height of the image. It represents the change in the height of the object after the reflection.
The formula for magnification in a mirror is –
Since, the magnification is positive, the image is virtual and since it is lesser than one, it is diminished.
Therefore, The image is formed at a distance of 8.57 m from the pole and it is virtual and diminished.
Note: The students should exercise extreme caution while substituting the values of u and v in the equations. As you can see, in the above steps, the value of u is substituted with entire brackets first, and then, solved by removing the brackets. This is the best practice to avoid errors due to sign convention in these problems.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
EMI starts from ₹2,775 per month
Recently Updated Pages
How to find Oxidation Number - Important Concepts for JEE

How Electromagnetic Waves are Formed - Important Concepts for JEE

Electrical Resistance - Important Concepts and Tips for JEE

Average Atomic Mass - Important Concepts and Tips for JEE

Chemical Equation - Important Concepts and Tips for JEE

Concept of CP and CV of Gas - Important Concepts and Tips for JEE

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Degree of Dissociation and Its Formula With Solved Example for JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

Dual Nature of Radiation and Matter Class 12 Notes: CBSE Physics Chapter 11

Formula for number of images formed by two plane mirrors class 12 physics JEE_Main

Electrical Field of Charged Spherical Shell - JEE




