Question

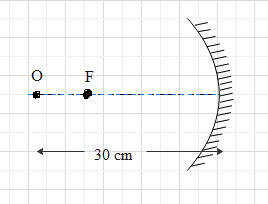
An object is present on the principal axis of a concave mirror at a distance \[30{\text{ }}cm\] from it. Focal length of the mirror is \[20{\text{ }}cm\]. If object starts moving with \[2{\text{ }}cm/s\] perpendicular to principal axis above axis, then:

A) image moves with velocity \[4{\text{ }}cm/s\] below the principal axis
B) image moves with velocity \[4{\text{ }}cm/s\] above the principal axis
C) image moves with velocity \[8{\text{ }}cm/s\] below principal axis
D) image moves with velocity \[8{\text{ }}cm/s\] above the principal axis.

Answer 1

Hint: We can use the mirror formula for the concave mirror and then change it into the magnification formula of the mirror. The velocity of the image can be determined by the product of the velocity of the object and magnification of the mirror.

Complete step by step answer:
We know that for a mirror, the mirror formula is given by-
$\dfrac{1}{v} + \dfrac{1}{u} = \dfrac{1}{f}$
In the given question, a concave mirror is given. An object is present at point \[O\] at a distance of \[30cm\]. The focal length of the mirror is \[20cm\]. So, the focus point is \[F\] which is \[20cm\] from the mirror. For concave mirror, we know that $u = - u$, $f = - f$, then the mirror formula becomes,
$\Rightarrow \dfrac{1}{v} + \dfrac{1}{{( - u)}} = \dfrac{1}{{( - f)}}$
or we can write the above equation as-
$\Rightarrow \dfrac{1}{v} - \dfrac{1}{u} = \dfrac{{ - 1}}{f}$
So, the equation becomes-
$\Rightarrow v = \dfrac{{fu}}{{u - f}}$
We can change the above equation into a magnification formula. So, the magnification formula is given by,
$\Rightarrow \dfrac{v}{u} = \dfrac{f}{{u - f}}$
Again, we know that if magnification of a mirror and the height of the object is given then the velocity of the image can be found. So, the formula for the velocity of the image is given by,
\[{\text{Velocity of image = }}\dfrac{{\partial {\text{(height of object)}}}}{{\partial {\text{t}}}}{{ \times }}\dfrac{{\text{f}}}{{{\text{f - u}}}}\]
According to the question, $f = 20cm$, $u = 10cm$ and \[\dfrac{{\partial {\text{(height of object)}}}}{{\partial {\text{t}}}} = 2cm/s\]. So, putting the values in the above equation we get,
$
  \Rightarrow {\text{Velocity of image = }}2{{ \times }}\dfrac{{20}}{{{\text{ - (20 - 10)}}}} \\
   \Rightarrow \text{Velocity of image = 2} \times \dfrac{{20}}{{ - 10}} \\
   \Rightarrow \text{Velocity of image = - 4cm/s} \\
$
The velocity of the image is \[4{\text{ }}cm/s\] which is greater than \[0\]. So, the image is moving downwards.

Hence the image is moving below the principal axis with a velocity of \[4{\text{ }}cm/s\]. Therefore, option (A) is correct.

Note:
If the velocity of the image is greater than \[0\] then the image is moving downwards and if the velocity of the image is less than \[0\] then the image is moving upwards. For a concave mirror, the distance of the object \[u\] and focal length \[f\] should be taken negatively. For convex mirror focal length \[f\] is always positive.