Question

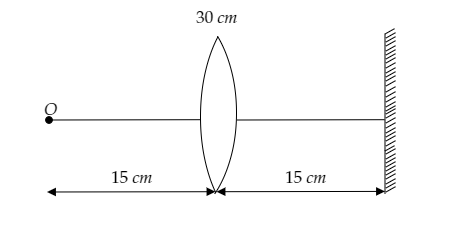
An object O is kept in front of a converging lens of a focal length $30{\text{cm}}$ behind which a plane mirror is kept at $15{\text{cm}}$ from the lens. Which of the following options is correct?

A) The final image is formed at $60{\text{cm}}$ from the lens towards the right of it.
B) The final image is formed at $60{\text{cm}}$ from the lens towards the left of it.
C) The final image is real.
D) The final image is virtual.

Answer 1

Hint:Here the object is placed at some distance from the lens. The image formed will be due to the refraction of light rays from the object at the lens. This first image will then act as the object for the plane mirror placed behind the lens. The image then formed of this object will be the final image formed. To find the image distance for the lens we use the thin lens equation.

Formula used:
-The thin lens equation is given by, $\dfrac{1}{v} - \dfrac{1}{u} = \dfrac{1}{f}$ where $v$ is the image distance, $u$ is the object distance and $f$ is the focal length of the lens.

Complete step by step solution.
Step 1: List the parameters given in the question.
The object is placed at a distance ${u_l} = 15{\text{cm}}$ from the lens to its left.
The focal length of the converging lens is given to be $f = 30{\text{cm}}$ .
A plane mirror is also placed to the right of the converging lens at a distance of $d = 15{\text{cm}}$ .

Step 2: Using the thin lens equation obtains the image distance of the first image formed by the lens.
The thin lens equation is given by, $\dfrac{1}{{{v_l}}} - \dfrac{1}{{{u_l}}} = \dfrac{1}{f}$
where ${v_l}$ is the image distance, ${u_l}$ is the object distance and $f$ is the focal length of the lens.
$ \Rightarrow \dfrac{1}{{{v_l}}} = \dfrac{1}{f} + \dfrac{1}{{{u_l}}}$ --------- (1)
Substituting for $f = 30{\text{cm}}$ and ${u_l} = - 15{\text{cm}}$in equation (1) we get, $\dfrac{1}{{{v_l}}} = \dfrac{1}{{30}} - \dfrac{1}{{15}} = \dfrac{{ - 1}}{{30}}$
$ \Rightarrow {v_l} = - 30{\text{cm}}$
Thus the first image ${I_1}$ formed by the converging lens will be at a distance ${v_l} = - 30{\text{cm}}$ and it is formed on the same side as the object $O$ is placed.
The ray diagram of the formation of the image ${I_1}$ is given below.

This image ${I_1}$ will now serve as the object for the plane mirror.

Step 3: Using the properties of the plane mirror, obtain the distance at which the final image or the second image is formed.
The object distance for the plane mirror will be ${u_m} = {v_l} + d$ ------- (2) in front of the plane mirror. (We neglect the sign).
Substituting for ${v_l} = 30{\text{cm}}$ and $d = 15{\text{cm}}$ in equation (2) we get ${u_m} = 30 + 15 = 45{\text{cm}}$ .
So the object is at a distance of ${u_m} = 45{\text{cm}}$ in front of the mirror.
For a plane mirror, the image formed will be at a distance equal to the distance at which the object is placed and will be on the other side of the plane mirror.
So the image distance of the plane mirror is ${v_m} = 45{\text{cm}}$ on its right or behind it.
So the location of the final image will be $v = {v_m} + d = 45 + 15 = 60{\text{cm}}$ to the right of the lens as the distance between the lens and the mirror is $d = 15{\text{cm}}$ .
So the correct option is A.
The ray diagram of the formation of the final image ${I_2}$ is given below.

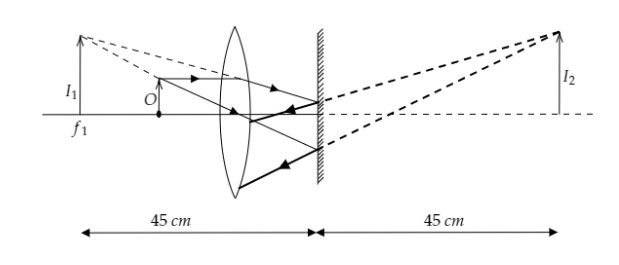
The refracted rays from the lens get reflected by the plane mirror. Then retracing the reflected rays will form the image behind the mirror. So the image formed is virtual.
So option D is also correct.

Thus the correct options are A and D.

Note:By sign convention, the distances to the left of the lens are taken to be negative and those to the right of the lens are taken to be positive. So we substitute ${u_l} = - 15{\text{cm}}$ in equation (1). However, the focal length of a converging lens is always positive. The first image is formed at the first focal point of the converging lens. The final image ${I_2}$ formed by the mirror will also be of the same height as its object ${I_1}$ .