Question

An open umbrella is held upright and rotated about the handle at a uniform rate of $21$ revolution in $44\,\,s$. If the rim of the umbrella is a circle of $100\,\,cm$ diameter and the height of the rim above ground is $150\,\,cm$, then the drops of water spinning off the rim will hit the ground from centre of umbrella, at a distance of:

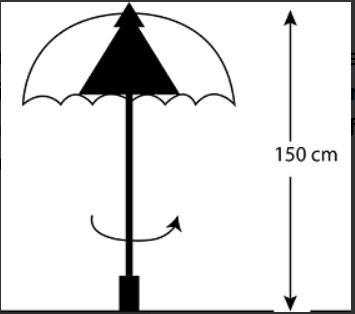
A) $200\,\,cm$
B) $52\,\,cm$
C) $172\,\,cm$
D) $97\,\,cm$

Answer 1

Hint:- In the given problem the distance of the water droplet can be calculated using the formula derived from the Pythagoras theorem for the triangles, which incorporates the radius of the water droplet and the range of the water droplet from the rim of the umbrella.

Useful formula:
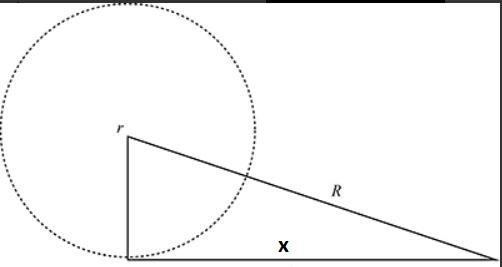
The drop of water that will hit the ground at a distance is given by;
$R = \sqrt {{r^2} + {x^2}} $
Where,$R$ denotes the distance travelled by the drop of water from the centre of the umbrella, $r$ denotes the radius of the water drop, $x$ denotes the distance from the centre of the umbrella.

Complete step by step solution:
The data given in the problem are:
Frequency of the umbrella is, $f = \dfrac{{21\,\,rev}}{{44\,\,s}}$,
Diameter of the rim of the umbrella is, $d = 100\,\,cm$,
Height of the rim above the ground, $h = 150\,\,cm$,

The velocity of the water drop is given by;
$v = r\omega $
Where, $v$ denotes the velocity by which the water drop falls, $r$ denoted the radius of the rim of the umbrella, $\omega $ denotes the angular speed by which the water drop fall from the umbrella.
Since,$\omega = 2\pi f$
$v = r \times 2\pi f$
Substitute the values of radius of the rim of the umbrella and the frequency;
By converting diameter into radius $r = 50\,\,cm$,
                    $r = 0.5\,\,m$.
$
  v = 0.5\,\,m \times 2 \times \pi \times \dfrac{{21}}{{44}} \\
  v = 0.5 \times 2.9 \\
  v = 1.5\,\,m\,{s^{ - 1}} \\
 $
The time taken by the water droplet to reach the ground is;
$t = \sqrt {\dfrac{{2h}}{g}} $
Where,$t$ denotes the time taken, $h$ denotes the height of the rim above the ground, $g$ denotes the acceleration due to gravity.
Substitute the values of height and gravity;
We know that $g = 9.8\,\,m{s^{ - 2}}$, \[h = 1.5\,\,m\];
$t = \sqrt {\dfrac{{2 \times 1.5}}{{9.8}}} $
On simplifying the above equation, we get;
$
  t = \sqrt {\dfrac{3}{{9.8}}} \\
  t = \sqrt {0.306125} \\
 $
On taking square root we get;
$t = 0.55\,\,s$
The range by which the water drops from the umbrella is given by,
$x = vt$
Where, $x$ denotes the range, $v$ denotes the velocity, $t$ denotes the time taken.
Substitute the values for velocity and time taken;
$x = 1.5\,\,m{s^{ - 1}} \times 0.55\,\,s$
On simplifying we get;
$x = 0.83\,\,m$
 The distance travelled by the drop of water when it hits the ground;
$R = \sqrt {{r^2} + {x^2}} $
Substitute the values of radius and range;
$
  R = \sqrt {{{\left( {0.5} \right)}^2} + {{\left( {0.83} \right)}^2}} \\
  R = \sqrt {0.25 + 0.6889} \\
  R = \sqrt {0.9389} \\
 $
On taking square root we get;
$R = 0.97\,\,m$
$R = 97\,\,cm$

Therefore, the distance travelled by the water drop from the centre of the umbrella I to the ground is $R = 97\,\,cm$.

Hence the option (D), $R = 97\,\,cm$ is the correct answer.

Note: In the problem that we solved we have converted from $cm$ to $m$ in order to get a standard value for the distance of the water drops, since $m$ is the standard unit and the found value is converted back in terms of $cm$ since it is the unit it is asked in the problem.