
Angle of dip
A.
B.
C.
D.

Answer
501.6k+ views
Hint: Using the formula for magnetic field find the magnetic field at position r. Then, find the magnetic field at
Formula used:
Complete answer:
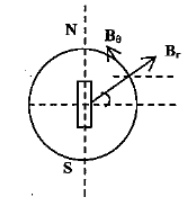
Consider the situation for dipoles at positions, r and
The magnetic field at position r is given by,
The magnetic field at
But,
Thus, substituting the equation. (3) in equation. (2) we get,
Similarly. Equation. (2) becomes,
Dividing equation.(4) by (5) we get,
Or we can also write it as,
Hence, the correct answer is option D i.e.
Note:
Here, the angle of dip means the angle of magnetic dip. Magnetic dip is defined as an angle which is made between the Earth’s magnetic field lines and horizontal plane. This angle is not constant. It depends on the point that is taken into consideration. And by latitude, we mean magnetic latitude. It is different from geographic latitude. It is defined with respect to the magnetic dipoles.
Formula used:
Complete answer:
Consider the situation for dipoles at positions, r and
The magnetic field at position r is given by,
The magnetic field at
But,
Thus, substituting the equation. (3) in equation. (2) we get,
Similarly. Equation. (2) becomes,
Dividing equation.(4) by (5) we get,
Or we can also write it as,
Hence, the correct answer is option D i.e.
Note:
Here, the angle of dip means the angle of magnetic dip. Magnetic dip is defined as an angle which is made between the Earth’s magnetic field lines and horizontal plane. This angle is not constant. It depends on the point that is taken into consideration. And by latitude, we mean magnetic latitude. It is different from geographic latitude. It is defined with respect to the magnetic dipoles.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




