Question

What are called the centre of curvature and radius of curvature of the mirror?

Answer 1

Hint: A mirror is a piece of glass that reflects light. When focussed via the lens of the eye or a camera, light that bounces off a mirror reflects a picture of whatever is in front of it. Mirrors reflect light in an equal but opposite direction, reversing the direction of the picture. This allows the spectator to see themselves or things behind them, as well as objects that are in their field of view but are at an angle from them, such as behind a corner.

Complete answer:
The centre of curvature of a curve is located at a position on the normal vector that is a distance from the curve equal to the radius of curvature. If the curvature is zero, it is the point at infinity.

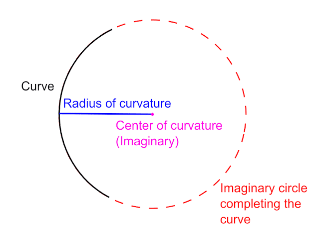
The osculating circle is located at the curve's centre of curvature. The junction point of two infinitely near normal lines to the curve was designated by Cauchy as the centre of curvature C.

The evolute of the curve is made up of the locus of centres of curvature for each point on the curve. The term "lens and mirrors" is commonly used in physics to refer to the study of lenses and mirrors. The radius of curvature, R, is the reciprocal of the curvature in differential geometry. It is equal to the radius of the circular arc that best approximates the curve at that point for a curve. The radius of curvature of a surface is the radius of a circle that best matches a normal section or combinations of normal sections.

Note: The circle, which has a curvature equal to the reciprocal of its radius, is the canonical example of a curve. Curvature is higher in smaller circles because they bend more sharply. The curvature of a differentiable curve's osculating circle, or the circle that best approximates the curve at this point, is the curvature of the curve. A straight line has zero curvature. In contrast to the tangent, which is a vector quantity, the curvature at a point is usually a scalar quantity, which means it may be represented as a single real number.