
Why are tetrahedral complexes generally high spin?
Answer
401.7k+ views
1 likes
Hint: For this, we should know about crystal field splitting in tetrahedral complexes. Same like octahedral crystal field, tetrahedral crystal field splits their orbitals into same
Complete answer:
A regular tetrahedron is similar to a cube. One atom at the centre of the cube and four of the eight corners of the cube contain ligands as shown in the following figure:
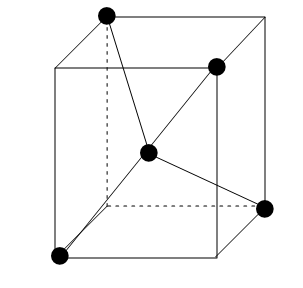
The direction
Due to which the angle between an
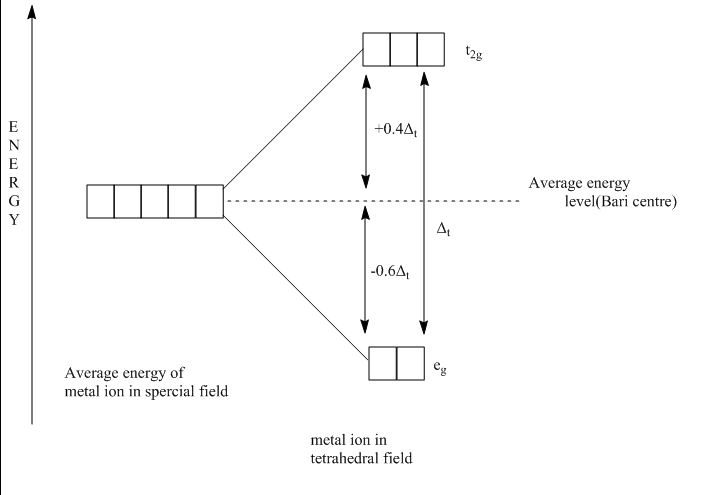
The
Note:
For coordination number four, the geometry for high spin is the tetrahedral as it has
Complete answer:
A regular tetrahedron is similar to a cube. One atom at the centre of the cube and four of the eight corners of the cube contain ligands as shown in the following figure:

The direction

Due to which the angle between an

The
Note:
For coordination number four, the geometry for high spin is the tetrahedral as it has
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE




