
How is average rate of change related to slope?
Answer
468.9k+ views
Hint: Slope is just a way of measuring the inclination of a line or the steepness of the line. It is defined by, change in
Average rate of change
Complete step-by-step solution:
Let’s assume the coordinates of those points as follows:
Let us consider the two points
Average rate of change
Now, substitute the two points in the formula of average rate of change
Average rate of change
Adding the two terms,
Simplifying the terms,
Average rate of change
Slope is calculated by finding the ratio of the vertical change to horizontal change between two distinct points on a line. The coordinates points to point a graph. And then to draw a straight line through the two points. Slope is something also referred to as the rate of change.
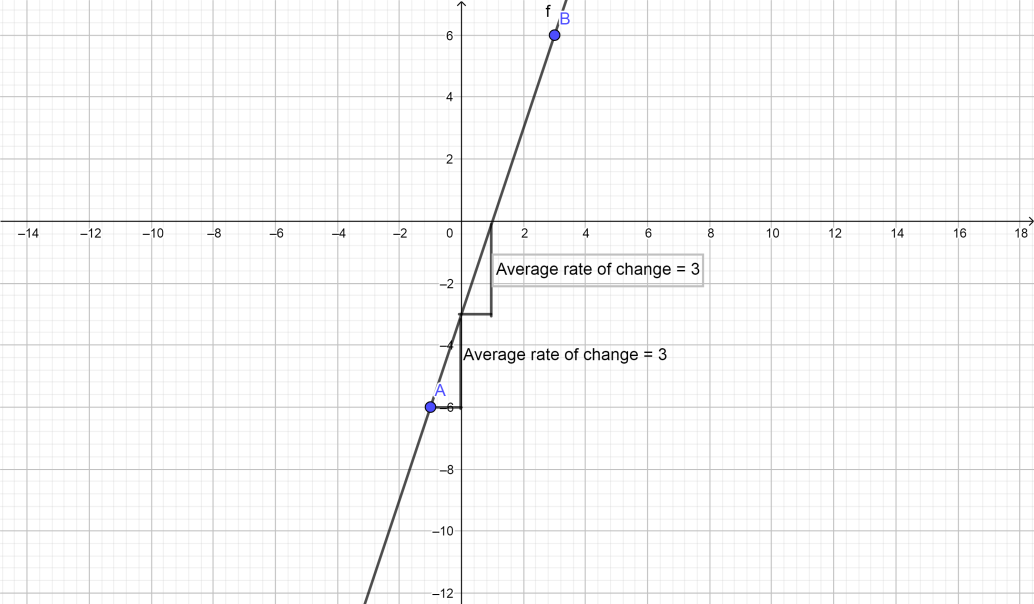
When we moved
Note: The rate of change between two points on either side of x becomes closer to the slope of x as the distance between the two points is reduced. When we work with functions, the average rate of change is expressed using function notation.
For the function is,
Average rate of change
Average rate of change
Complete step-by-step solution:
Let’s assume the coordinates of those points as follows:
Let us consider the two points
Average rate of change
Now, substitute the two points in the formula of average rate of change
Average rate of change
Adding the two terms,
Simplifying the terms,
Average rate of change
Slope is calculated by finding the ratio of the vertical change to horizontal change between two distinct points on a line. The coordinates points to point a graph. And then to draw a straight line through the two points. Slope is something also referred to as the rate of change.

When we moved
Note: The rate of change between two points on either side of x becomes closer to the slope of x as the distance between the two points is reduced. When we work with functions, the average rate of change is expressed using function notation.
For the function is,
Average rate of change
Latest Vedantu courses for you
Grade 7 | CBSE | SCHOOL | English
Vedantu 7 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
EMI starts from ₹3,775 per month
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE




