
What is black body radiation? Explain the characteristics of it.
Answer
408.6k+ views
1 likes
Hint: A black body is an idealized object that absorbs all electromagnetic radiation it comes in contact with. It then emits the absorbed radiation as thermal radiation in a continuous spectrum according to its temperature. It follows certain laws when it emits thermal radiation.
Formula used:
Complete step-by-step answer:
The radiation emitted by a black body is called black body radiation. The distribution of energy of a black body radiation
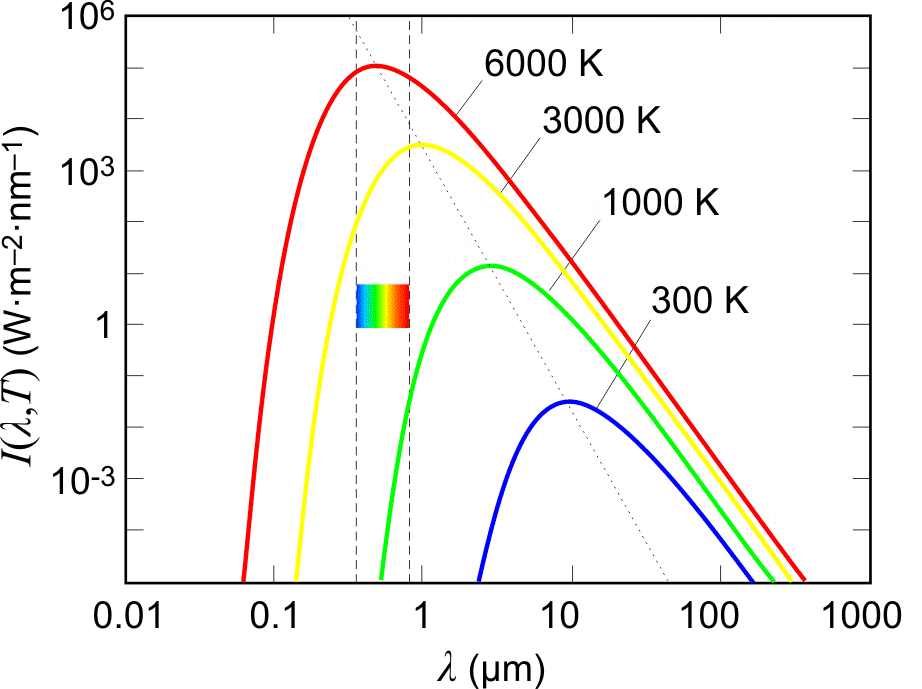
Characteristics of a blackbody radiation spectra are:
(1) The emissive power of a blackbody
(2) Each curve has a characteristic form with a maximum for
(3)
(4) The area under each curve gives the total radiant power per unit area
Note: The Stefan-Boltzmann constant,
Formula used:
Complete step-by-step answer:
The radiation emitted by a black body is called black body radiation. The distribution of energy of a black body radiation

Characteristics of a blackbody radiation spectra are:
(1) The emissive power of a blackbody
(2) Each curve has a characteristic form with a maximum for
(3)
(4) The area under each curve gives the total radiant power per unit area
Note: The Stefan-Boltzmann constant,
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




