
Body diagonal of a cube is 866 pm. Its edge length would be:
(A) 408 pm
(B) 1000 pm
(C) 500 pm
(D) 600 pm
Answer
494.7k+ views
Hint: In geometry a space diagonal of a polyhedron is a line connecting two vertices that are not on the same face.
Write the relationship between the body diagonal ‘d’ and the edge length ‘a’ of a cube:
Complete answer:
Consider the right angled triangle marked with blue colour,
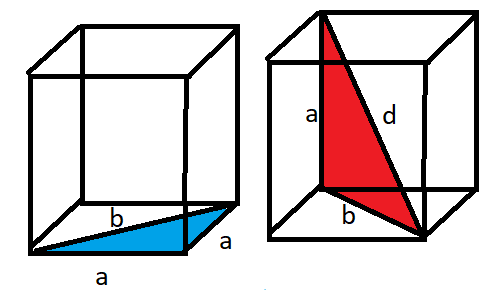
Apply Pythagoras theorem and calculate the length of the hypotenuse.
Now consider the right angled triangle marked with blue colour
Apply Pythagoras theorem and calculate the length of the hypotenuse.
Write the relationship between the body diagonal ‘d’ and the edge length ‘a’ of a cube:
Rearrange the above equation to obtain the expression for the edge length:
Body diagonal of a cube is
Substitute
Thus, the edge length of the cube would be 500 pm.
Hence, the correct option is the option (C).
Note: During the derivation of the relationship between the body diagonal ‘d’ and the edge length ‘a’ of a cube, Pythagoras theorem is used. According to this theorem, for a right angled triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the remaining two sides.
Write the relationship between the body diagonal ‘d’ and the edge length ‘a’ of a cube:
Complete answer:
Consider the right angled triangle marked with blue colour,

Apply Pythagoras theorem and calculate the length of the hypotenuse.
Now consider the right angled triangle marked with blue colour
Apply Pythagoras theorem and calculate the length of the hypotenuse.
Write the relationship between the body diagonal ‘d’ and the edge length ‘a’ of a cube:
Rearrange the above equation to obtain the expression for the edge length:
Body diagonal of a cube is
Substitute
Thus, the edge length of the cube would be 500 pm.
Hence, the correct option is the option (C).
Note: During the derivation of the relationship between the body diagonal ‘d’ and the edge length ‘a’ of a cube, Pythagoras theorem is used. According to this theorem, for a right angled triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the remaining two sides.
Recently Updated Pages
Basicity of sulphurous acid and sulphuric acid are

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




