Question

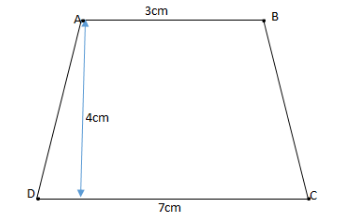
Calculate the area of the isosceles trapezium drawn below,

Answer 1

Hint: We can solve this by two easy methods. First method is by using the rectangles. Second method is by using the standard formula of area of trapezium. We know that the area of trapezium is \[ = \dfrac{1}{2}(a + b)d\] , where \[a\] and \[b\] is the length of the two parallel sides, \[d\] is the perpendicular distance between two parallel sides.

Complete step-by-step answer:
Let’s see the second method.
We have a formula for finding the area of trapezium \[ = \dfrac{1}{2}(a + b)d\] .
From the given diagram we have \[a = 3\] , \[b = 7\] and \[d = 4\] . Substituting we have,
 \[ = \dfrac{1}{2}(a + b)d\]
 \[ = \dfrac{1}{2} \times (3 + 7) \times 4\]
By BODMAS we solve the calculation part in brackets, we get:
 \[ = \dfrac{1}{2} \times 10 \times 4\]
Multiplying,
 \[ = \dfrac{1}{2} \times 40\]
Dividing we have,
 \[ = 20\;c{m^2}\]
Hence, the area of given isosceles trapezium is \[20\;c{m^2}\] .
                                               OR
Let’s see the first method. The given trapezium is formed from a rectangle of equal area.
Sum of the lengths of that rectangle is equal to the sum of the parallel sides of the trapezium.
 \[ = 7 + 3\]
 \[ = 10\;cm\]
Length of that rectangle \[ = \dfrac{{10}}{2}\]
 \[ = 5\;cm\]
Height of that rectangle is equal to the height of the trapezium \[ = 4\;cm\] .
Area f rectangle = Area of trapezium \[ = 5 \times 4\]
 \[ = 20\;c{m^2}\]
Hence, the area of given isosceles trapezium is \[20\;c{m^2}\]
So, the correct answer is “ \[20\;c{m^2}\] ”.

Note: We use a second method which is easy to solve and direct substitution. Since the area is in square units. Given the length of sides are in centimetre, hence we have area in square centimetre. An isosceles trapezoid is a trapezoid in which the base angles are equal and therefore the left and right side lengths are also equal. This concept is used in solving the first method. We can also solve this by dividing into two triangles which is a bit complicated so use the above method to solve this type of problem.