
Calculate the equivalent resistance between the points A and B for the following combination of resistors:
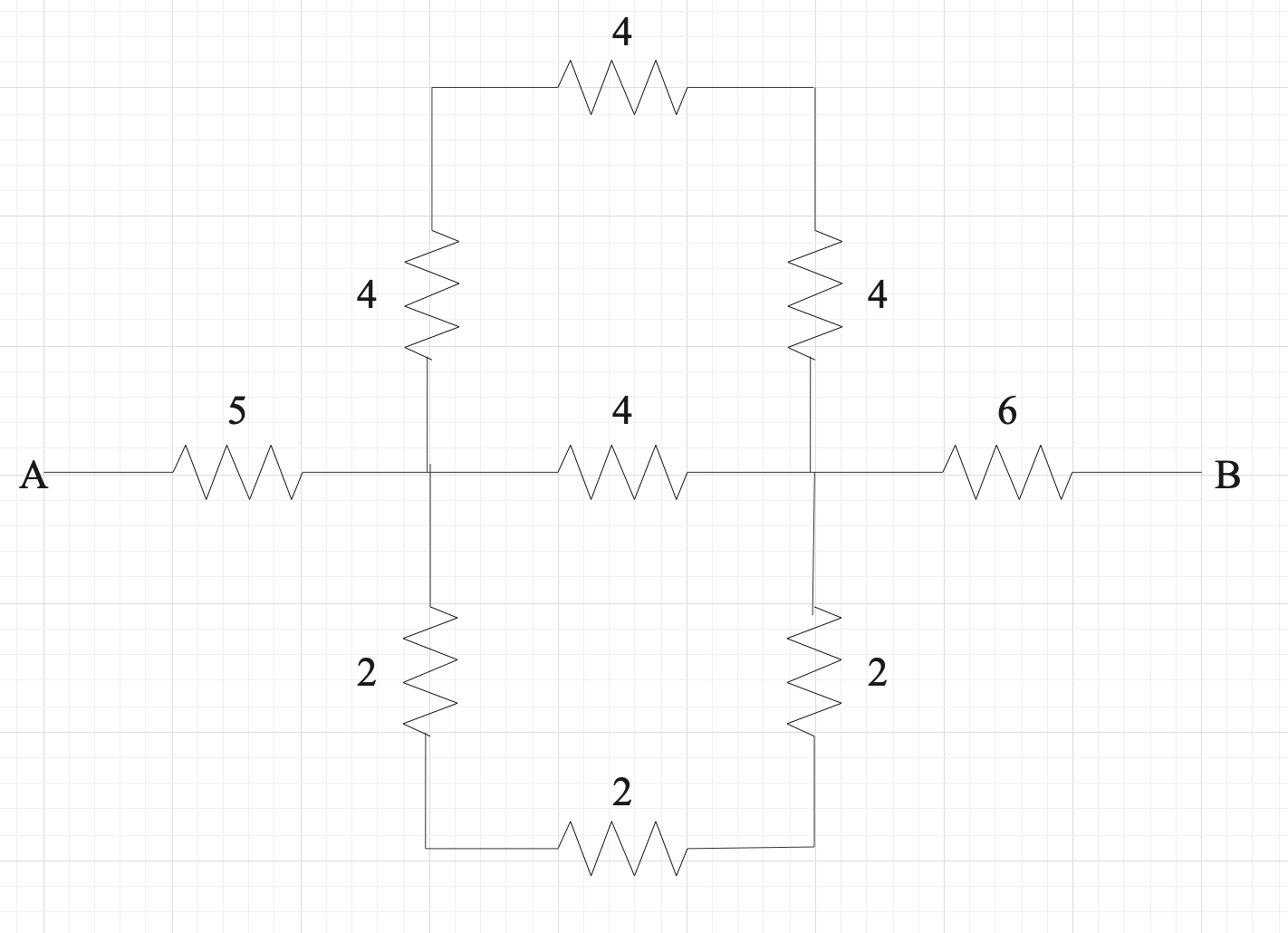

Answer
507.3k+ views
Hint: First find the series connections, then solve the parallel connection. Divide the circuit into three segments. Find the equivalent resistance for the middle segment using parallel and series connection formulas. Then calculate the final equivalent resistance using the simple series formula.
Formula Used:
The equivalent resistance of 3 parallel resistors is given by,
The equivalent resistance of 3 series resistors is given by,
Where,
Complete step by step answer:
First, let us look at the circuit diagram,
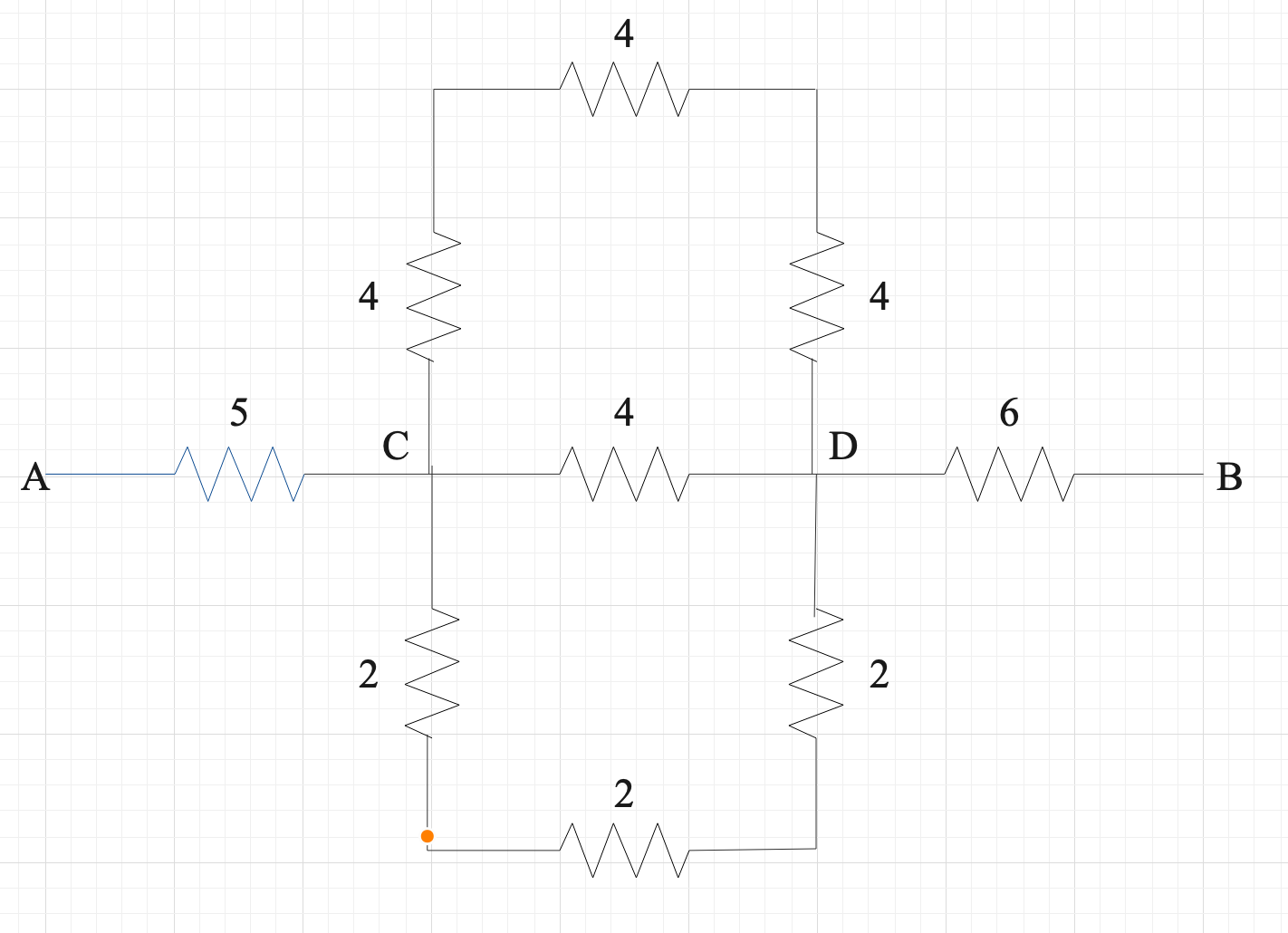
For simplicity, we can divide the circuit diagram into three segments,
AC, CD, and DB.
First, let us solve the CD segment.
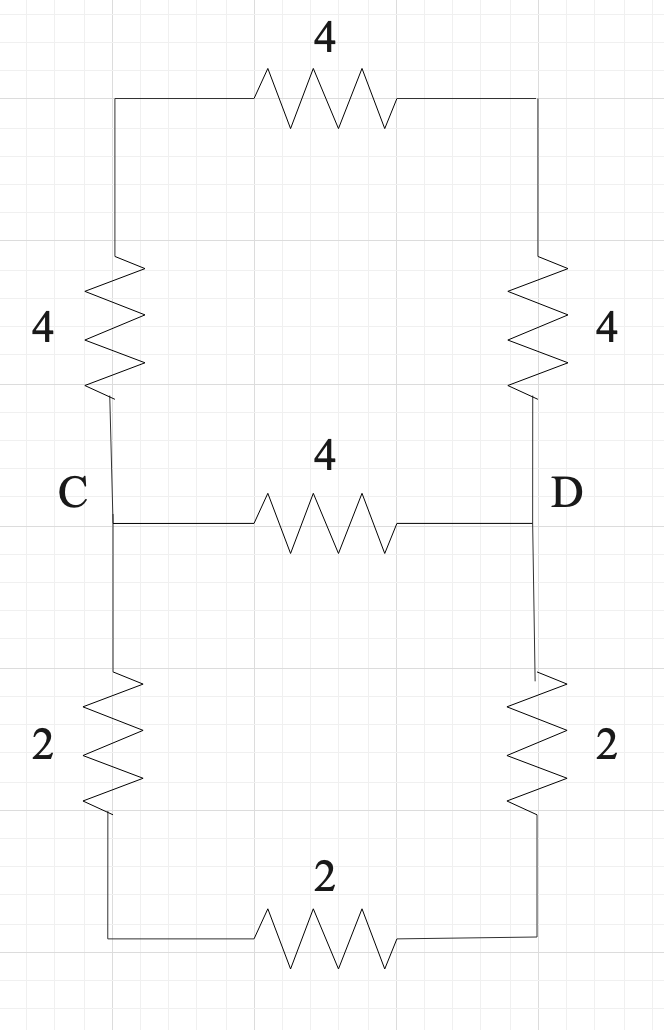
So, the 4Ω resistances are connected in series, so the equivalent resistance of the top branch is -
(4+4+4) Ω = 12Ω
Now, the equivalent resistance of the bottom branch is -
(2+2+2) Ω = 6Ω
So, the CD segment should look like this:
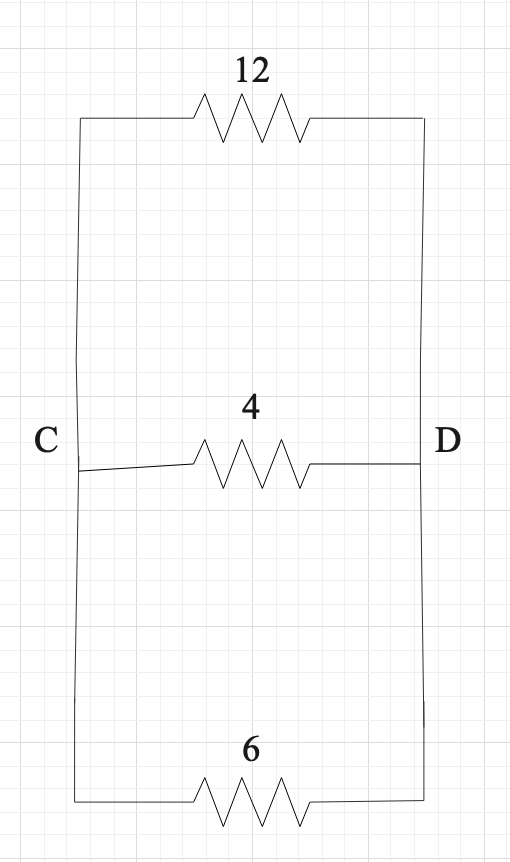
Now we use equation (1) to get the equivalent resistance of the resistance system.
The equivalent resistance of the CD segment is,
Hence the overall circuit diagram should like this,
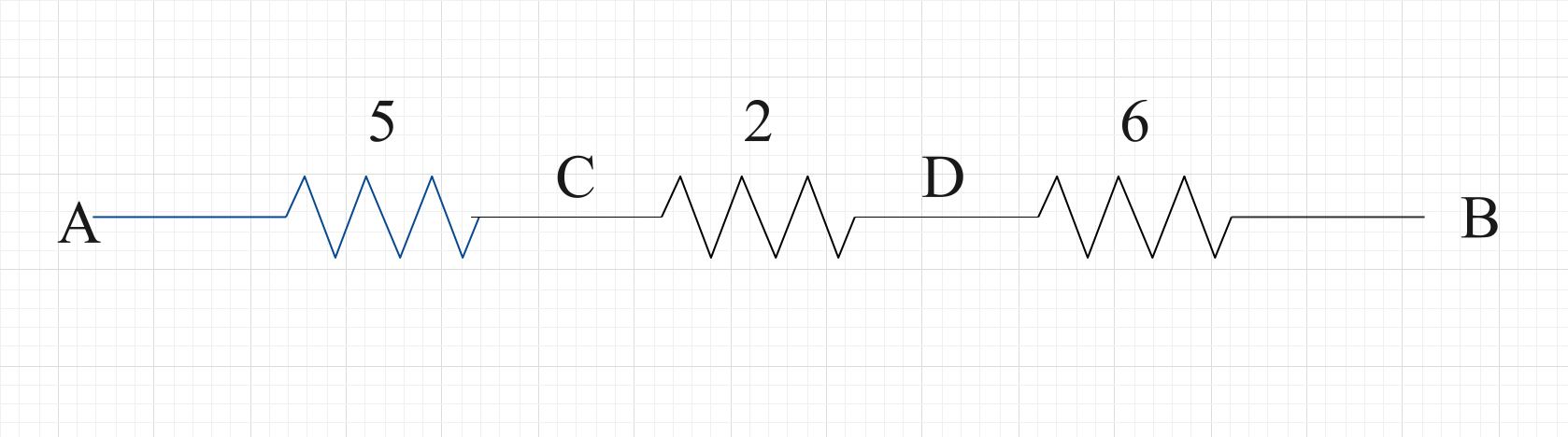
This is a simple series connection. So, the equivalent resistance of the system is,
The equivalent resistance between points A and B = 13 Ω
Note: We have used a complex formula for the parallel system. You can use the simple formula shown below to calculate the equivalent resistance of the system.
Where,
You can use equation (3) to calculate the equivalent resistance of the parallel system. The calculation will be less in some cases.
Formula Used:
The equivalent resistance of 3 parallel resistors is given by,
The equivalent resistance of 3 series resistors is given by,
Where,
Complete step by step answer:
First, let us look at the circuit diagram,

For simplicity, we can divide the circuit diagram into three segments,
AC, CD, and DB.
First, let us solve the CD segment.

So, the 4Ω resistances are connected in series, so the equivalent resistance of the top branch is -
(4+4+4) Ω = 12Ω
Now, the equivalent resistance of the bottom branch is -
(2+2+2) Ω = 6Ω
So, the CD segment should look like this:

Now we use equation (1) to get the equivalent resistance of the resistance system.
The equivalent resistance of the CD segment is,
Hence the overall circuit diagram should like this,

This is a simple series connection. So, the equivalent resistance of the system is,
The equivalent resistance between points A and B = 13 Ω
Note: We have used a complex formula for the parallel system. You can use the simple formula shown below to calculate the equivalent resistance of the system.
Where,
You can use equation (3) to calculate the equivalent resistance of the parallel system. The calculation will be less in some cases.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




