
Calculate the packing efficiency of a simple cubic cell?
Answer
479.7k+ views
3 likes
Hint:
The packing efficiency can be defined as the ratio of the volume of a single atom in a unit cell to the volume of the entire unit cell multiplied by
FORMULA USED:
Where
Complete answer:
The cubic unit cell is the simplest form of packing seen in a simple cubic structure.The packing efficiency of the simple cubic lattice can be found based on the length of the edge. The edge length can be derived as follows:
First, we have to consider the following image: we can see that the halves of two atoms lie along the edge of the cube. This means that if we consider the radius of each atom to be
Here
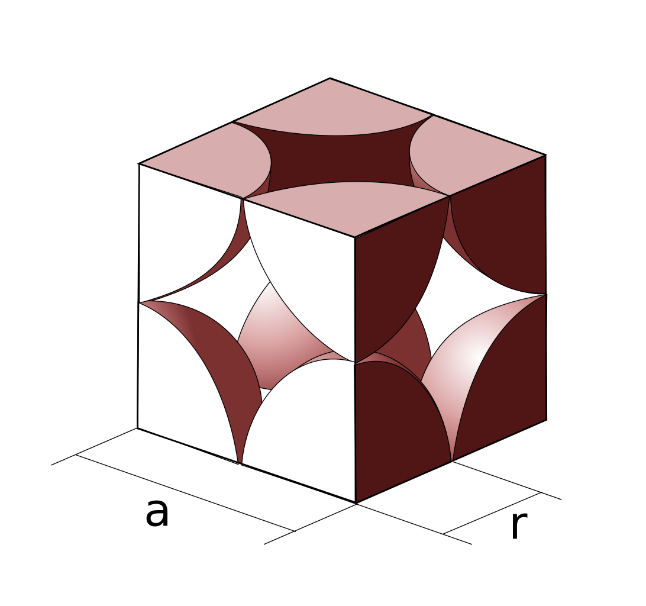
Using the formula mentioned above it will now be easy to derive the packing efficiency of this lattice. This is demonstrated below:
we have to plug the value for edge length.
Since
cancelling the multiples of
After multiplying with
Therefore, the packing efficiency of the simple cubic lattice is
Note: The
It is also imperative to remember the edge length of different crystal lattices as they differ as in face centered, body centered and others.
The packing efficiency can be defined as the ratio of the volume of a single atom in a unit cell to the volume of the entire unit cell multiplied by
FORMULA USED:
Where
Complete answer:
The cubic unit cell is the simplest form of packing seen in a simple cubic structure.The packing efficiency of the simple cubic lattice can be found based on the length of the edge. The edge length can be derived as follows:
First, we have to consider the following image: we can see that the halves of two atoms lie along the edge of the cube. This means that if we consider the radius of each atom to be
Here

Using the formula mentioned above it will now be easy to derive the packing efficiency of this lattice. This is demonstrated below:
we have to plug the value for edge length.
Since
cancelling the multiples of
After multiplying with
Therefore, the packing efficiency of the simple cubic lattice is
Note: The
It is also imperative to remember the edge length of different crystal lattices as they differ as in face centered, body centered and others.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
EMI starts from ₹3,487.34 per month
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




