
Can your lower quartile be the same as your median in a box and whisker plot? How?
Answer
439.5k+ views
Hint: To solve this question first we need to know about the box and whisker plot.
Complete step by step solution:
We know that a box plot is a graph that offers us an idea about how the values in the data should be spread. Box plot extends its lines from the boxes generally called as whiskers. Whiskers are used to indicate variability outside the upper and the lower quartile.
A box plot has a five number summary of a set of data that includes the minimum score, first quartile (lower), median, third quartile (upper) and maximum score. The lower quartile falls below 25 percent of scores and upper quartile falls below 75 percent of the scores.
Let us take an example of a dataset.
We have a sorted data as
Now, the median
So we get the lower quartile of the dataset as
Now, the third quartile or upper quartile of the dataset is
Now, the five numbers summary of a set of data is
Minimum score = 1
First quartile (lower)
Median
Third quartile (upper)
Maximum score = 7
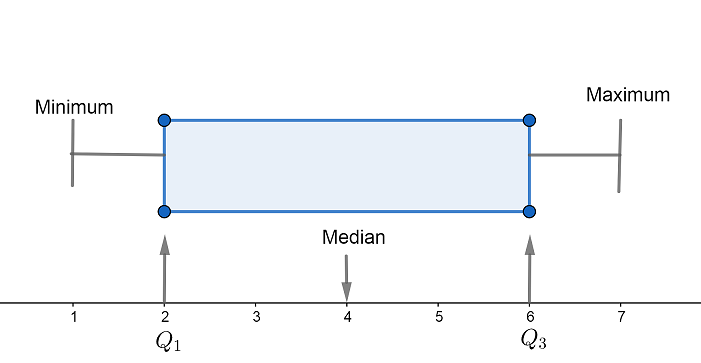
In this case we didn’t get the lower quartile the same as the median.
If we take the dataset as
Then we will get the five numbers summary of a set of data as
Minimum score = 1
First quartile (lower)
Median
Third quartile (upper)
Maximum score = 4
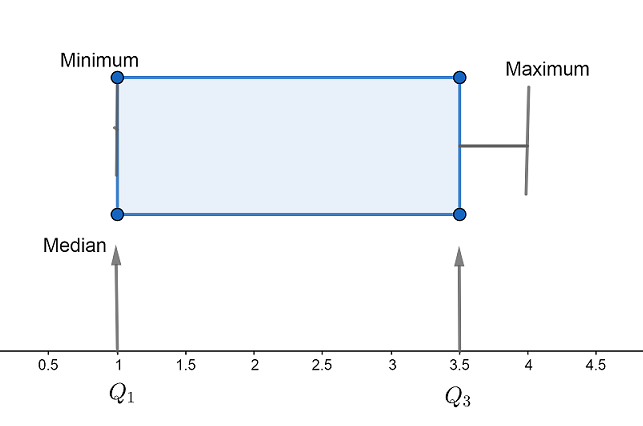
In this case we get the lower quartile same as the median in a box and whisker plot.
Note:
The point to be noted is that the lower quartile is the median of the lower half of the dataset. The whiskers go from each quartile to the minimum or maximum. A vertical line goes through the box at the median.
Complete step by step solution:
We know that a box plot is a graph that offers us an idea about how the values in the data should be spread. Box plot extends its lines from the boxes generally called as whiskers. Whiskers are used to indicate variability outside the upper and the lower quartile.
A box plot has a five number summary of a set of data that includes the minimum score, first quartile (lower), median, third quartile (upper) and maximum score. The lower quartile falls below 25 percent of scores and upper quartile falls below 75 percent of the scores.
Let us take an example of a dataset.
We have a sorted data as
Now, the median
So we get the lower quartile of the dataset as
Now, the third quartile or upper quartile of the dataset is
Now, the five numbers summary of a set of data is
Minimum score = 1
First quartile (lower)
Median
Third quartile (upper)
Maximum score = 7

In this case we didn’t get the lower quartile the same as the median.
If we take the dataset as
Then we will get the five numbers summary of a set of data as
Minimum score = 1
First quartile (lower)
Median
Third quartile (upper)
Maximum score = 4

In this case we get the lower quartile same as the median in a box and whisker plot.
Note:
The point to be noted is that the lower quartile is the median of the lower half of the dataset. The whiskers go from each quartile to the minimum or maximum. A vertical line goes through the box at the median.
Recently Updated Pages
What percentage of the area in India is covered by class 10 social science CBSE

The area of a 6m wide road outside a garden in all class 10 maths CBSE

What is the electric flux through a cube of side 1 class 10 physics CBSE

If one root of x2 x k 0 maybe the square of the other class 10 maths CBSE

The radius and height of a cylinder are in the ratio class 10 maths CBSE

An almirah is sold for 5400 Rs after allowing a discount class 10 maths CBSE

Trending doubts
What did the military generals do How did their attitude class 10 english CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

For Frost what do fire and ice stand for Here are some class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Write a letter to the principal requesting him to grant class 10 english CBSE




