Question

Carla can use 100 square feet of floor space in her school’s gymnasium, in any way she chooses to set up a computer station for a science fair. She has chosen to use floor space in the shape of a rectangle, with dimensions that are the whole number.
Draw all possible rectangles with an area of square feet and whole number dimensions.

Answer 1

Hint: In this question, we need to form different rectangles with an area of 100 square feet. Since, $\text{Area}=\text{Length}\times \text{Breadth}$ so we need to find possible length and breadth which could be multiplied to get a product as 100. For this, we will find different factor pairs such that their product is 100.

Complete step-by-step answer:
Here, we need to find different length and breadth such that their sum is 100. For this, let us find factor pairs of 100. And then draw a rectangle for each corresponding length and breadth.
(i) Dividing 100 by 1 gives us 100. So length could be 1 feet and breadth could be 100 feet to form a rectangle of area 100 square feet.

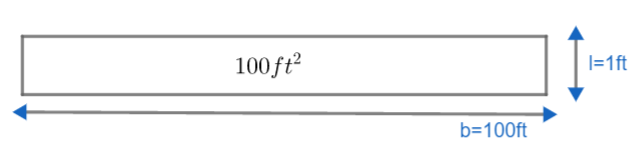
(ii) Dividing 100 by 2 gives us $\dfrac{100}{2}=50$. So length could be 2 feet and breadth could be 50 feet to form a rectangle of area 100 square feet.

(iii) Dividing 100 by 3 gives us $\dfrac{100}{3}=3.33$ which is not the whole number so this is not our required pair.
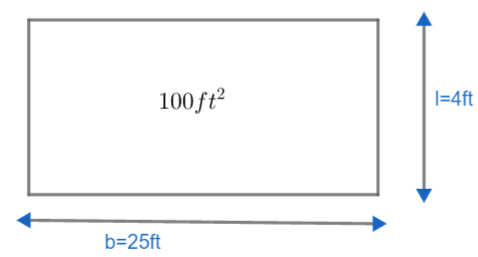
(iv) Dividing 100 by 4 gives us $\dfrac{100}{4}=25$. So length could be 4 feet and breadth could be 25 feet to form a rectangle with area 100 square feet.

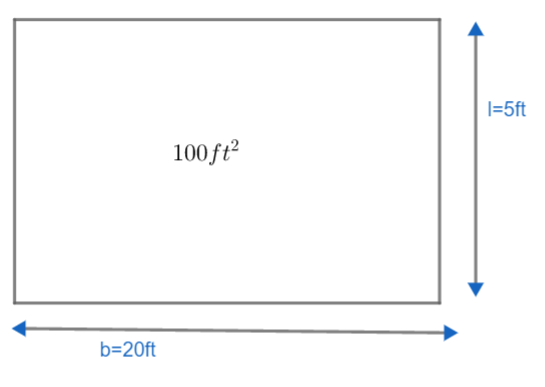
(v) Dividing 100 by 5 gives us $\dfrac{100}{5}=20$. So length could be 5 feet and breadth could be 20 feet to form a rectangle with area 100 square feet.

(vi) Since 100 is not divisible by 3, so it would not be divisible by 6. Dividing 100 by 7 gives us $\dfrac{100}{7}=14.2$ which is not the whole number. Dividing 100 by 8 gives us $\dfrac{100}{8}=12.5$ which is not a whole number. Since 100 is not divisible by 3, so it would not be divisible by 9.
Dividing 100 by 10 gives us $\dfrac{100}{10}=10$. So length could be 10 feet and breadth could be 10 feet to form a rectangle with area 100 square feet.

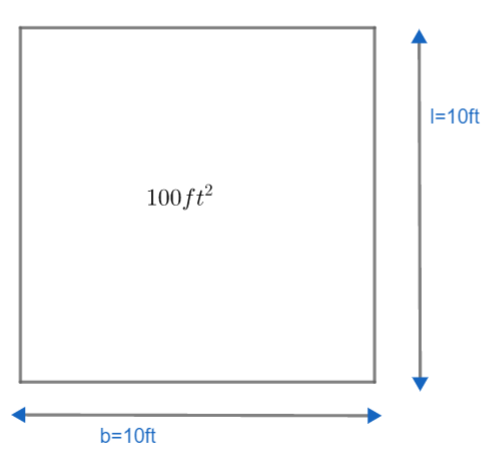
After that, numbers will be repeated so these are all the required rectangles.

Note: Students should note that, in the last rectangle we have length = breadth which makes it a square. We have included it because every square is also a rectangle. Make sure both length and breadth are whole numbers. Squared units are used for area. Since the area was in square feet so our length and breadth should be in feet.