
Consider a concavo-convex lens of glass of refractive index
A)
B)
C)
D)

Answer
496.2k+ views
Hint:The processes involved in this arrangement include refraction of the incident ray at the water- glass interface followed by the reflection of the refracted ray by the silvered convex surface and finally refraction at the glass-water interface. The light rays incident on the convex surface of the lens are reflected since the convex surface is coated with silver i.e., the convex surface acts as a mirror.
Complete step by step answer.
Step 1: List the data provided in the question.
Given, the radius of curvature of the convex surface is 20 cm and that of the concave surface is 60 cm.
Also given, the refractive index of glass is
A total of three processes take place in this arrangement – refraction, reflection and again refraction.
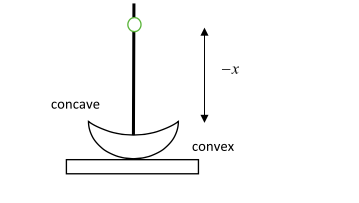
Initially, we consider the case where water is not present on the concave surface of the lens. Then the light from the object gets refracted at the air-glass interface. The refracted ray then gets reflected by the convex surface which is coated by silver (acts as a concave mirror). The reflected ray then gets refracted now at the glass-air interface and an image is formed. The position of the pin is placed so that its image coincides with it.
When the concave surface is filled with water the position of the image formed shifts and it no longer coincides with the position of the pin. So our aim is to find the distance through which the pin has to be moved to obtain its coinciding image when water is filled in the concave surface of the lens.
Step 2: Find the position of the image formed by the refraction at the air-glass interface.
Let
Then for refraction, we use the expression
Substituting for
we get,
Simplifying the above equation gives us
or,
Simplifying the above equation we get,
Equation (2) represents the position of the image formed by refraction.
This image formed at
Step 3: Find the position of the image formed by reflection from the silver-coated convex surface.
The silver-coated convex surface acts as a concave mirror and its focal length
Now for reflection, the mirror equation is given by,
Let the position of the image formed by reflection be
Substituting these values in equation (3), we get
The above equation is simplified as
Finally, we have
This image formed at
Step 4: Find the position of the image formed by the refraction at the glass-air interface.
Now,
Then for refraction, we use the expression
Substituting for
we get,
Simplifying the above equation gives us
or,
i.e.,
Solving the above equation we get,
Thus the position of the pin is placed at a distance
Now the concave part of the lens is filled with water. Thus the image of the pin no longer coincides with it.
Step 5: Sketch a diagram for the setup where the concave surface is filled with water. Find the position of the image formed by refraction between the water-glass interface.
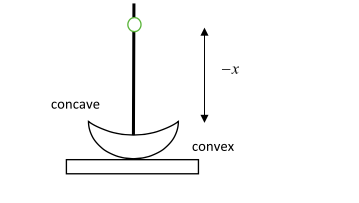
Let
Then for refraction, we use the expression
Substituting for
we get,
Simplifying the above equation we have
or,
Simplifying the above equation we get,
Equation (6) represents the position of the image formed by refraction.
This image formed at
Step 6: Find the position of the image formed by reflection at the silver-coated convex surface.
The silver-coated convex surface acts as a concave mirror and its focal length will be
Now for reflection, the mirror equation is given by,
Let the position of the image formed by reflection be
Substituting these values in equation (7), we get
The above equation is simplified as
Finally, we have
This image formed at
Step 7: Find the position of the image formed by the refraction at the glass-water interface.
Now,
Then for refraction, we use the expression
Substituting for
we get,
Simplifying the above equation gives us
or,
i.e.,
Solving the above equation we get,
Thus the position of the pin is placed at a distance
Step 8: Calculate the distance through which the pin is moved when the concave surface is filled with water.
Initially, we found that when the pin is placed at a distance
When the concave surface was filled with water, the pin had to be placed at a distance
Therefore, when water is added to the concave surface, the pin must be moved through a distance
Note: When representing any measure of distance, sign convention must be applied. By sign convention, the position of the object is negative for both the concave mirror and concave lens. The position of the image is negative for a concave lens but it is positive for a concave mirror. The focal length is negative for a concave mirror and concave lens.
Complete step by step answer.
Step 1: List the data provided in the question.
Given, the radius of curvature of the convex surface is 20 cm and that of the concave surface is 60 cm.
Also given, the refractive index of glass is
A total of three processes take place in this arrangement – refraction, reflection and again refraction.
Initially, we consider the case where water is not present on the concave surface of the lens. Then the light from the object gets refracted at the air-glass interface. The refracted ray then gets reflected by the convex surface which is coated by silver (acts as a concave mirror). The reflected ray then gets refracted now at the glass-air interface and an image is formed. The position of the pin is placed so that its image coincides with it.
When the concave surface is filled with water the position of the image formed shifts and it no longer coincides with the position of the pin. So our aim is to find the distance through which the pin has to be moved to obtain its coinciding image when water is filled in the concave surface of the lens.
Step 2: Find the position of the image formed by the refraction at the air-glass interface.
Let
Then for refraction, we use the expression
Substituting for
we get,
Simplifying the above equation gives us
or,
Simplifying the above equation we get,
Equation (2) represents the position of the image formed by refraction.
This image formed at
Step 3: Find the position of the image formed by reflection from the silver-coated convex surface.
The silver-coated convex surface acts as a concave mirror and its focal length
Now for reflection, the mirror equation is given by,
Let the position of the image formed by reflection be
Substituting these values in equation (3), we get
The above equation is simplified as
Finally, we have
This image formed at
Step 4: Find the position of the image formed by the refraction at the glass-air interface.
Now,
Then for refraction, we use the expression
Substituting for
we get,
Simplifying the above equation gives us
or,
i.e.,
Solving the above equation we get,
Thus the position of the pin is placed at a distance
Now the concave part of the lens is filled with water. Thus the image of the pin no longer coincides with it.
Step 5: Sketch a diagram for the setup where the concave surface is filled with water. Find the position of the image formed by refraction between the water-glass interface.

Let
Then for refraction, we use the expression
Substituting for
we get,
Simplifying the above equation we have
or,
Simplifying the above equation we get,
Equation (6) represents the position of the image formed by refraction.
This image formed at
Step 6: Find the position of the image formed by reflection at the silver-coated convex surface.
The silver-coated convex surface acts as a concave mirror and its focal length will be
Now for reflection, the mirror equation is given by,
Let the position of the image formed by reflection be
Substituting these values in equation (7), we get
The above equation is simplified as
Finally, we have
This image formed at
Step 7: Find the position of the image formed by the refraction at the glass-water interface.
Now,
Then for refraction, we use the expression
Substituting for
we get,
Simplifying the above equation gives us
or,
i.e.,
Solving the above equation we get,
Thus the position of the pin is placed at a distance
Step 8: Calculate the distance through which the pin is moved when the concave surface is filled with water.
Initially, we found that when the pin is placed at a distance
When the concave surface was filled with water, the pin had to be placed at a distance
Therefore, when water is added to the concave surface, the pin must be moved through a distance
Note: When representing any measure of distance, sign convention must be applied. By sign convention, the position of the object is negative for both the concave mirror and concave lens. The position of the image is negative for a concave lens but it is positive for a concave mirror. The focal length is negative for a concave mirror and concave lens.
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




