
Consider a rectangle of length
Answer
489.6k+ views
Hint: For the above question, first consider a rectangle, its breadth and length after that directly apply the formula of area of rectangle and put all values , then calculate it to get the value of area.
Complete step-by-step answer:
To find the area of a given rectangle, let us consider a rectangle of length
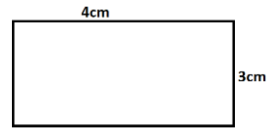
We also know that the area of the rectangle is a product of its length and breadth.
Therefore,
Area of rectangle = length
Area of rectangle=
Now
Area of rectangle=
It is the required area of the rectangle.
Hence option A is the correct option.
Note: Rectangle is one of the types of quadrilaterals, which have opposite sides equal and parallel. Also all angles are equal to
Complete step-by-step answer:
To find the area of a given rectangle, let us consider a rectangle of length

We also know that the area of the rectangle is a product of its length and breadth.
Therefore,
Area of rectangle = length
Area of rectangle=
Now
Area of rectangle=
It is the required area of the rectangle.
Hence option A is the correct option.
Note: Rectangle is one of the types of quadrilaterals, which have opposite sides equal and parallel. Also all angles are equal to
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
How many ounces are in 500 mL class 8 maths CBSE

10 slogans on organ donation class 8 english CBSE

The LCM and HCF of two rational numbers are equal Then class 8 maths CBSE

How many ten lakhs are in one crore-class-8-maths-CBSE

The minimum age required for becoming the Prime Minister class 8 social science CBSE

How was Junagadh State merged into the Indian Unio class 8 social science CBSE





