
Consider an ellipse having major axis and minor axis parallel to x-axis and y-axis respectively. Its foci S and S’ one
1.) Eccentricity of ellipse is:
a)
b)
c)
d) None
2.) Length of major axis is:
a)
b)
c)
d)
Answer
492k+ views
Hint: To solve this problem we first calculate lengths of perpendicular distance from given foci, as the product of perpendicular distance from foci is equal to square of length of minor axis. After this we calculate distance between two foci as it is always equal to
Distance formula between two points:
Complete step-by-step answer:
Let consider an ellipse whose major and minor axis are parallel to x-axis and y-axis.
And let ‘a’ and ‘b’ are semi major and minor axis of ellipse.
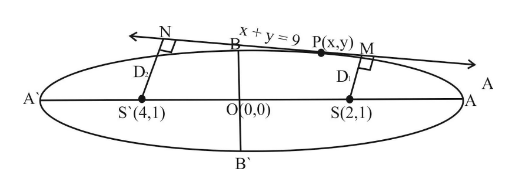
Also, foci of ellipse are given as
Since, we know that the product of perpendicular distance from the foci on tangent line is equal to square of semi major axis.
Now, calculating
Also,
Using above calculated values of
Also, we know that distance between foci of an ellipse is equal to ‘
Therefore,
Now, squaring both side we have
Also, we know that there is a relation between eccentricity and minor, major axes. Which is given as
Substituting above calculated values in above formed equation.
Hence, from above we see that the eccentricity of the given ellipse is
Therefore, from given four options for problem 1.) the correct option is (b).
Now, to find the length of the major axis.
From above we have a relation which is
Or
Substituting value of ‘e’ from above we have:
Where, ‘a’ is the length of the semi major axis of ellipse.
Therefore, length of major axis of ellipse is given as =
Hence, length of major axis =
So, the correct answer is “Option 2 AND D”.
Note: Ellipse is a symmetrical figure. So, while calculation we can consider either ‘a’ or ‘b’ as major and minor axis or vice-versa result remains the same. Which implies that while using the eccentricity formula of major axis of ellipse or minor axis of ellipse wouldn’t make an effect on calculation and hence on result.
Distance formula between two points:
Complete step-by-step answer:
Let consider an ellipse whose major and minor axis are parallel to x-axis and y-axis.
And let ‘a’ and ‘b’ are semi major and minor axis of ellipse.

Also, foci of ellipse are given as
Since, we know that the product of perpendicular distance from the foci on tangent line is equal to square of semi major axis.
Now, calculating
Also,
Using above calculated values of
Also, we know that distance between foci of an ellipse is equal to ‘
Therefore,
Now, squaring both side we have
Also, we know that there is a relation between eccentricity and minor, major axes. Which is given as
Substituting above calculated values in above formed equation.
Hence, from above we see that the eccentricity of the given ellipse is
Therefore, from given four options for problem 1.) the correct option is (b).
Now, to find the length of the major axis.
From above we have a relation which is
Or
Substituting value of ‘e’ from above we have:
Where, ‘a’ is the length of the semi major axis of ellipse.
Therefore, length of major axis of ellipse is given as =
Hence, length of major axis =
So, the correct answer is “Option 2 AND D”.
Note: Ellipse is a symmetrical figure. So, while calculation we can consider either ‘a’ or ‘b’ as major and minor axis or vice-versa result remains the same. Which implies that while using the eccentricity formula of major axis of ellipse or minor axis of ellipse wouldn’t make an effect on calculation and hence on result.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




