Question

Construct a square of side $5cm$ and find its area?

Answer 1

Hint: Assume that the square's name is ABCD. Consider AB, which has a length of $5cm$ . Draw the square using the help of a ruler and compass, using the properties of squares that all sides are of equal length and each angle equals ${90^ \circ }$ .

Complete step-by-step solution:
We're supposed to make a square here. The square's side length is listed as $5cm$ .
We now know that a square is a quadrilateral with the following properties:

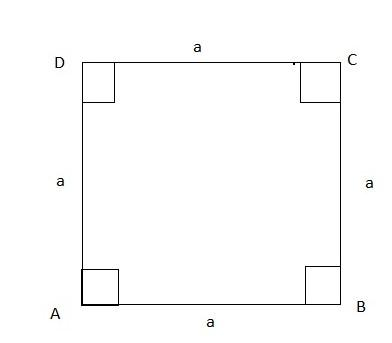
A square's sides are all the same length. Each angle is ${90^ \circ }$ in length. A square's diagonals AC and BD intersect each other at ${90^ \circ }$ .
We will now construct a square ABCD with $5cm$ on each side.
We will follow these steps.
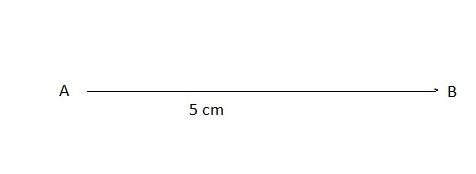
 We will draw a line segment AB with a length of $5cm$ using a ruler.

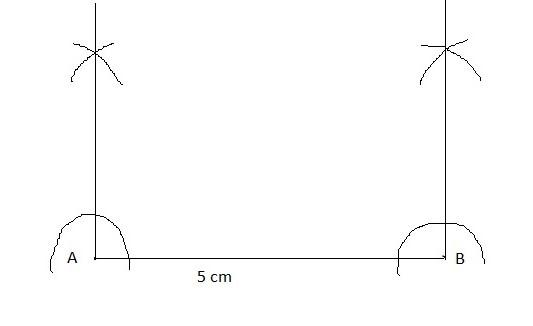
We will draw perpendicular lines between points A and B by placing the compass at point A, draw an arc, and bisect the ${180^ \circ }$angle. We will carry on with point B in the same way and then extend these parallel lines.

We will now cut a $5cm$ length of perpendicular from point A and mark it as D. Similarly cut a length of $5cm$ on its perpendicular from point B and mark it as C.

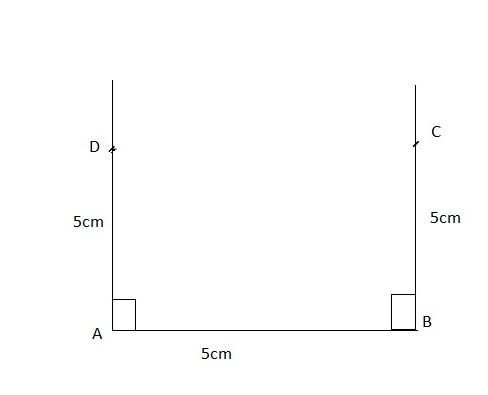
We will join C and D to produce a line segment CD.

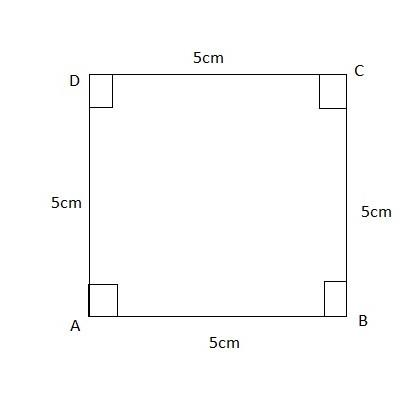
Hence, our construction is complete.
We will calculate the area of square
=$side \times side$
$ = 5 \times 5c{m^2}$
$ = 25c{m^2}$
Area of square having each side 5cm is 25 square cm.

Note: Because these are typical shapes whose constructions are requested, it is important to learn the properties of basic quadrilaterals such as the parallelogram, square, rectangle, and rhombus. To compose the above question, you must memorise all of the properties of squares. Because it is not stated that we must use a compass, you may construct a \[90\]-degree angle using a protractor.