
Answer
389.7k+ views
Hint:Given is the measure of the right angle. Then we will first draw the rough figure of the respective construction. After that we will draw the base as AC. Then we will mark a right angle at point or say vertex C. Now we have to draw the other side of the triangle. Since the given triangle is isosceles the other side will be of the same measurement. So in a compass we will take the distance and mark the other side. The base point and the marked point will make the triangle after joining.
Complete step by step answer:
The given question is related to construction. For that first we will draw the rough figure.
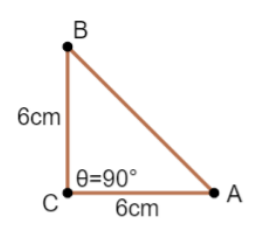
Now we will construct this triangle step by step.
Step1: draw the base of the triangle first like the given $A$C is 6 cm.
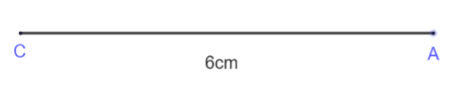
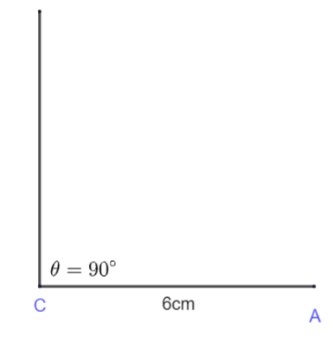
Now we will draw an angle.
Step 2: keep the center of the protractor on the point $C$ and measure a right angle and mark the point. Then draw the line with the help of the ruler.
Now on the line drawn perpendicular to the point $C$ or line $AC$ we will mark an arc at a distance of 6 cm. This can be done with the help of a compass. Then mark the point as the third vertex of the triangle as $B$.
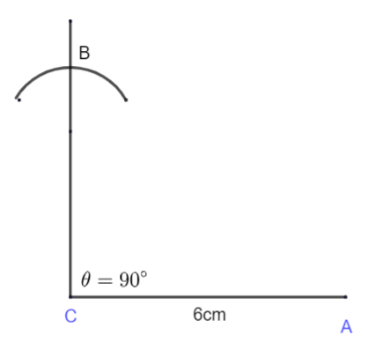
Now join point $A$ and $B$. this is the third side of the triangle.
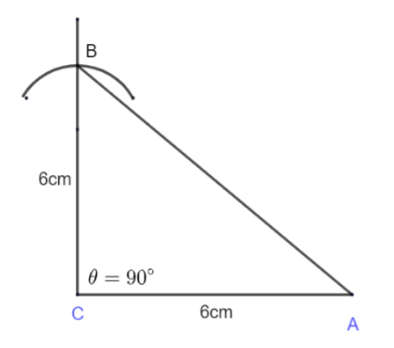
Now this is the given isosceles right angled triangle.
Note: The triangle is given as isosceles, that is the reason we have taken two sides of the same length and need not measure the length of the hypotenuse. But when any two sides are given then find the third side using Pythagoras theorem such that \[hypt = \sqrt {bas{e^2} + heigh{t^2}} \]. Then either the side can be found or the hypotenuse. But the two sides that are base and height will always be perpendicular to each other.
Complete step by step answer:
The given question is related to construction. For that first we will draw the rough figure.

Now we will construct this triangle step by step.
Step1: draw the base of the triangle first like the given $A$C is 6 cm.

Now we will draw an angle.
Step 2: keep the center of the protractor on the point $C$ and measure a right angle and mark the point. Then draw the line with the help of the ruler.

Now on the line drawn perpendicular to the point $C$ or line $AC$ we will mark an arc at a distance of 6 cm. This can be done with the help of a compass. Then mark the point as the third vertex of the triangle as $B$.

Now join point $A$ and $B$. this is the third side of the triangle.

Now this is the given isosceles right angled triangle.
Note: The triangle is given as isosceles, that is the reason we have taken two sides of the same length and need not measure the length of the hypotenuse. But when any two sides are given then find the third side using Pythagoras theorem such that \[hypt = \sqrt {bas{e^2} + heigh{t^2}} \]. Then either the side can be found or the hypotenuse. But the two sides that are base and height will always be perpendicular to each other.
Recently Updated Pages
10 Examples of Evaporation in Daily Life with Explanations

10 Examples of Diffusion in Everyday Life

1 g of dry green algae absorb 47 times 10 3 moles of class 11 chemistry CBSE

If the coordinates of the points A B and C be 443 23 class 10 maths JEE_Main

If the mean of the set of numbers x1x2xn is bar x then class 10 maths JEE_Main

What is the meaning of celestial class 10 social science CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Which are the Top 10 Largest Countries of the World?

How do you graph the function fx 4x class 9 maths CBSE

Who was the leader of the Bolshevik Party A Leon Trotsky class 9 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Which is the largest saltwater lake in India A Chilika class 8 social science CBSE

Ghatikas during the period of Satavahanas were aHospitals class 6 social science CBSE




